Question

How to prove that an exterior angle of a cyclic quadrilateral is equal to its opposite interior angle?

Answer 1

Hint:
Here, we will first construct a cyclic quadrilateral with the angles. Then by using the properties of the cyclic quadrilateral, we will get two equations. We will then equate the equations and simplify them further to prove that an exterior angle of a cyclic quadrilateral is equal to its opposite interior angle.

Complete step by step solution:
We will first draw the diagram based on the given information. Let ABCD be a cyclic quadrilateral. Now, let us consider a line segment DE extending from the base of the cyclic quadrilateral.

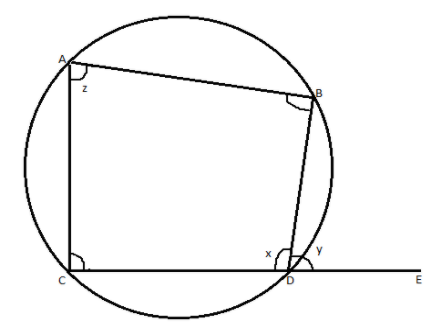
Now, we have to prove \[\angle BDE = \angle CAB\].
From the figure, we can say \[\angle BDE\] is an exterior angle of a cyclic quadrilateral. \[\angle CAB\] is an interior angle opposite to the exterior angle.
Now, let us consider \[\angle BDE = y\], \[\angle CDB = x\] and \[\angle BAC = z\]
We know that opposite angles of a cyclic quadrilateral are supplementary.
\[ \Rightarrow \angle CDB + \angle BAC = 180^\circ \]
Now, we get
\[ \Rightarrow x + z = 180^\circ \] ………………………………………..\[\left( 1 \right)\]
We know that adjacent angles in a straight angle are supplementary.
\[ \Rightarrow \angle CDB + \angle BDE = 180^\circ \]
Substituting the values from the figure, we get
\[ \Rightarrow x + y = 180^\circ \] …………………………………………………………………..\[\left( 2 \right)\]
Now, by equating the equations \[\left( 1 \right)\] and \[\left( 2 \right)\], we get
\[ \Rightarrow x + z = x + y\]
Subtracting \[x\] from both sides, we get
\[ \Rightarrow z = y\]
Thus, we get \[\angle BDE = \angle BAC\].

Therefore, an exterior angle of a cyclic quadrilateral is equal to its opposite interior angle which is also the property of a cyclic quadrilateral.

Note:
We know that a Quadrilateral is a four sided polygon. Cyclic Quadrilateral is a Quadrilateral which is inscribed on a circle and lies within the circumference of the circles. An angle formed by extending any one side of a polygon is called an exterior angle. Some of the properties of a cyclic quadrilateral are the sum of either pair of a opposite angles of a quadrilateral is \[180^\circ \] and if the sum of any pair of opposite angles of a quadrilateral is \[180^\circ \] then the quadrilateral is cyclic.