Question

Prove that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.

Answer 1

Hint: We construct the perpendiculars from points D and E to the opposite sides and calculate the areas of triangles using different heights. Take the ratio of the triangles and cancel the required terms.
* Area of a triangle is given by half of the product of its base and its height.

Complete step-by-step solution:
First we will draw any triangle ABC.

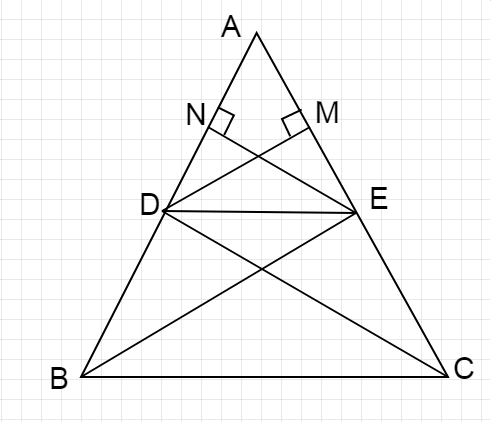
In this triangle line DE is drawn parallel two the side BC and intersects to the other two sides AB and AC at D and E respectively.
We have to show that, ${\displaystyle \frac{AD}{DB}}={\displaystyle \frac{AE}{EC}}$
For that we have to construct some lines.
Let us join BE and CD and then draw DM perpendicular to AC and EN perpendicular to AB.
To obtain the ratios we will consider three triangles such are$△ADE,△BDE,△\text{DEC}$.
Now, Area of$$△ADE={\displaystyle \frac{1}{2}}\times$$base $$\times$$height
$$\Rightarrow$$Area of$$△ADE={\displaystyle \frac{1}{2}}\times AD\times EN$$
Similarly, Area of$$△BDE={\displaystyle \frac{1}{2}}\times DB\times EN$$
For the other side AC:
Area of$$△ADE={\displaystyle \frac{1}{2}}\times AE\times D\mathrm{M}$$
And
Area of$$△DEC={\displaystyle \frac{1}{2}}\times \mathrm{E}C\times D\mathrm{M}$$
To obtain the ratios,
${\displaystyle \frac{ar\left(ADE\right)}{ar\left(BDE\right)}}={\displaystyle \frac{{\displaystyle \frac{1}{2}}\times AD\times EN}{{\displaystyle \frac{1}{2}}\times DB\times EN}}$
Here 1/2 and EN will get cancelled.
$\Rightarrow {\displaystyle \frac{ar\left(ADE\right)}{ar\left(BDE\right)}}={\displaystyle \frac{AD}{DB}}$ ………. 1)
Similarly,
${\displaystyle \frac{ar\left(ADE\right)}{ar\left(DEC\right)}}={\displaystyle \frac{{\displaystyle \frac{1}{2}}\times AE\times DM}{{\displaystyle \frac{1}{2}}\times EC\times DM}}$
$\Rightarrow {\displaystyle \frac{ar\left(ADE\right)}{ar\left(DEC\right)}}={\displaystyle \frac{AE}{EC}}$ ……… 2)
See, the triangles$△BDE$ &$△DEC$ are on the same base DE and between the same parallel lines BC and DE.
Hence, their areas will also be the same.
$\therefore ar\left(BDE\right)=ar\left(DEC\right)$ ………… 3)
Therefore from the statements (1), (2), (3) we can say,
${\displaystyle \frac{AD}{DB}}={\displaystyle \frac{AE}{EC}}$
Hence we proved that the other two sides divided in the same ratio.

Note: Many students make the mistake of calculating the areas of wrong triangles and end up taking the wrong ratio, keep in mind we have to take the ratio of those two triangles which give us the ratio of sides. Also, we can denote area of $△ADE$
$ar\left(ADE\right)$ and similarly we can write for any triangles.
Students should be familiar with the basic formulas like area of triangle, area of square, area of circle, area of rectangle.