
How do you prove that quadrilateral
Answer
469.5k+ views
Hint: A parallelogram is a quadrilateral in which the opposite sides are parallel and of equal length. To check whether a quadrilateral is a parallelogram or not. We have to check if its opposite sides are parallel. As we know that parallel sides have equal slopes, in other words, we have to check if the opposite sides have an equal slope or not. For any given two points,
Complete step-by-step answer:
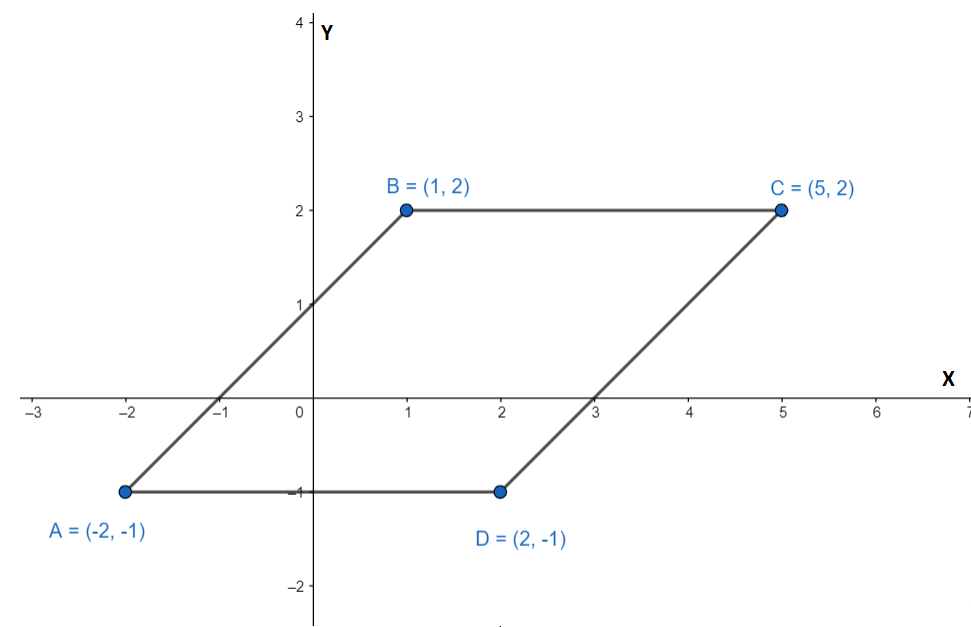
We are given the points
For line joining
For the line joining
For the line joining
For the line joining the
From the above values, we can say that
Hence the quadrilateral
We can also plot the points on the graph as follows,
Note: One should remember the properties of the sides, angles, diagonals of different types of quadrilaterals to solve these types of questions. Here if we calculate the length of the sides
Complete step-by-step answer:

We are given the points
For line joining
For the line joining
For the line joining
For the line joining the
From the above values, we can say that
Hence the quadrilateral
We can also plot the points on the graph as follows,
Note: One should remember the properties of the sides, angles, diagonals of different types of quadrilaterals to solve these types of questions. Here if we calculate the length of the sides
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




