Question

Prove that the median to the base of an isosceles triangle is perpendicular to the base.

Answer 1

Hint: Here first we will prove the two triangles formed by the median of isosceles triangle to be congruent and then with the help of linear pair property we will prove that the median is perpendicular to the base of the triangle.
The linear pair property states that the sum of two adjacent angles form \[{180^ \circ }\]

Complete step-by-step answer:
          

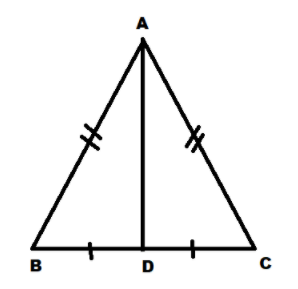
Let ABC is an isosceles triangle with AB=AC and let AD be the median to the base BC then D is the midpoint of BC.
Also ABC being an isosceles triangle implies:

\[\angle ABC = \angle ACB\]

In \[\Delta ABD\] and \[\Delta ACD\]

\[
  AB = AC\left( {{\text{equal sides}}} \right) \\
  \angle ABC = \angle ACB\left( {{\text{equal angles}}} \right) \\
  BD = DC\left( {{\text{BC bisected by median AD}}} \right) \\
 \]
Therefore, by SAS criterion of congruence triangles
\[\Delta ABD \cong \Delta ACD\]

Hence,
\[\angle ADB = \angle ADC\left( {{\text{by Corresponding parts of Congruent triangles}}} \right)\]

Let \[\angle ADC = \angle ADB = x\]

Now we know that by linear pair property states that the sum of two adjacent angles form \[{180^ \circ }\]
Hence,

\[\angle ADB + \angle ADC = {180^ \circ }\]

Putting in the values we get:-
\[
   \Rightarrow x + x = {180^ \circ } \\
   \Rightarrow 2x = {180^ \circ } \\
   \Rightarrow x = \dfrac{{{{180}^ \circ }}}{2} \\
   \Rightarrow x = {90^ \circ } \\
 \]

Hence, \[\angle ADC = \angle ADB = {90^ \circ }\]
Therefore AD is perpendicular to base BC.
Hence proved.

Note: An alternative method to solve this question is using the vector algebra.
               

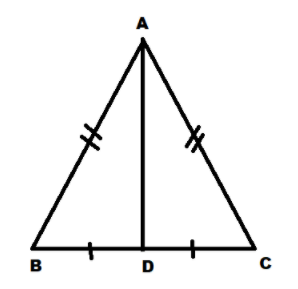
Take A as the origin and let \[\overrightarrow b \overrightarrow {,c} \] be the position vectors of B and C respectively with respect to the origin A such that \[\overrightarrow {AB} = \overrightarrow b \] and \[\overrightarrow {AC} = \overrightarrow c \]……………………………..(1)
Now since D is the midpoint of BC
Hence by midpoint formula the position vector of D with respect to A is:-
\[\overrightarrow {AD} = \dfrac{{\overrightarrow b + \overrightarrow c }}{2}\]
Also,
\[\overrightarrow {BC} = {\text{Position vector of C}} - {\text{Position vector of B}}\]
Hence putting the values we get:-
\[\overrightarrow {BC} = \overrightarrow c - \overrightarrow b \]
Now we know that the dot product of two perpendicular vectors is zero.
Hence we will check if the dot product of vectors \[\overrightarrow {AD} \] and \[\overrightarrow {BC} \] is zero or not.
Taking the dot product we get:-
\[
  \overrightarrow {AD} .\overrightarrow {BC} = \left( {\dfrac{{\overrightarrow b + \overrightarrow c }}{2}} \right).\left( {\overrightarrow c - \overrightarrow b } \right) \\
  \overrightarrow {AD} .\overrightarrow {BC} = \dfrac{1}{2}\left[ {\left( {\overrightarrow b + \overrightarrow c } \right).\left( {\overrightarrow c - \overrightarrow b } \right)} \right] \\
 \]
Simplifying it further we get:-
\[\overrightarrow {AD} .\overrightarrow {BC} = \dfrac{1}{2}\left[ {\overrightarrow b .\overrightarrow c - \overrightarrow b .\overrightarrow b + \overrightarrow c .\overrightarrow c - \overrightarrow c .\overrightarrow b } \right]\]
Now we know that:-
\[
  \overrightarrow b .\overrightarrow b = {\left| {\overrightarrow b } \right|^2} \\
  \overrightarrow c .\overrightarrow c = {\left| {\overrightarrow c } \right|^2} \\
  \overrightarrow b .\overrightarrow c = \overrightarrow c .\overrightarrow b \\
 \]
Therefore, putting in the values we get:-
\[\overrightarrow {AD} .\overrightarrow {BC} = \dfrac{1}{2}\left[ {{{\left| {\overrightarrow c } \right|}^2} - {{\left| {\overrightarrow b } \right|}^2}} \right]\]
Now substituting the values from equation 1 we get:-
\[\overrightarrow {AD} .\overrightarrow {BC} = \dfrac{1}{2}\left[ {A{C^2} - A{B^2}} \right]\]
Since ABC is an isosceles triangle
Therefore, \[AC = AB\]
Hence putting the values in above equation we get:-
\[
  \overrightarrow {AD} .\overrightarrow {BC} = \dfrac{1}{2}\left( 0 \right) \\
   \Rightarrow \overrightarrow {AD} .\overrightarrow {BC} = 0 \\
 \]
Now since the dot product of vectors \[\overrightarrow {AD} \] and \[\overrightarrow {BC} \] comes out be zero
Hence they are perpendicular to each other
Therefore the median AD is perpendicular to the base BC of the triangle
Hence proved.