
Prove that the time of flight 'T' and horizontal range 'R' of a projectile motion are connected by the equation
Answer
499.8k+ views
1 likes
Hint: Consider the projectile motion to be the sum of two rectilinear motion in x-axis and y-axis.
Apply equations of motion separately in these two motions:
Where, u = initial velocity, v = final velocity, s = displacement, a = acceleration and t = time.
Time of flight is the total time taken to complete the projectile motion, it will be double the time taken to reach the maximum height. Hence, calculate time to reach maximum height by using equation
And the horizontal range is the displacement of the projectile in x direction. Since, there is no acceleration in x direction so it can be simply calculated using equation
Complete step by step answer:
Let us consider a ball projected at an angle θ with respect to the horizontal x-axis with the initial velocity u as shown below:
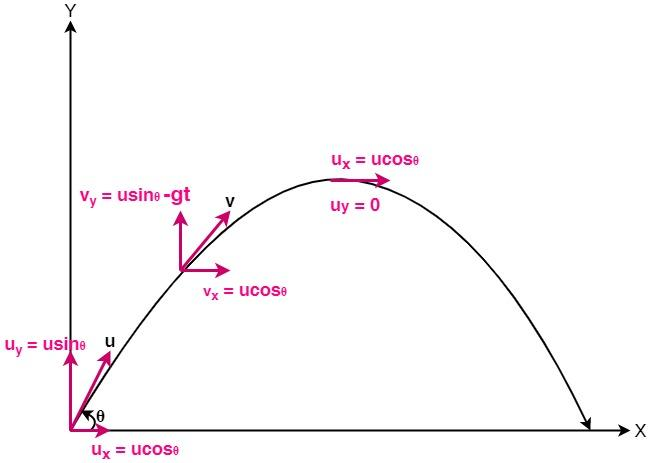
In a Projectile Motion, there are always two simultaneous independent rectilinear motions happening:
1. Along the x-axis: along x-axis velocity is uniform which is responsible for the horizontal (forward) motion of the particle.
2. Along y-axis: The particle is moving with a uniform acceleration but varying velocity which is responsible for the vertical (downwards) motion of the particle.
The x components and y components of the initial velocity can be expressed as:
Accelerations in the horizontal and vertical motions of the particle moving in projectile can be expressed as:
There is no acceleration in the horizontal motion of the particle moving in projectile.
The acceleration in the vertical motion of the particle in projectile is just due to acceleration due to gravity:
The velocity of the particle moving in projectile at any point in path of projectile can be given as:
The horizontal velocity remains constant,
But the vertical velocity varies linearly, because the acceleration is constant. At any time, t, the velocity is:
Now, finding:
1. Time of flight:
As we know velocity at max height, v = 0
Using:
Ball will take the same time to return to the ground.
Time of flight :
Horizontal Range = Horizontal Velocity x Flight Time
Hence, proved.
Note:
In projectile motion at any time t, the displacement components are given by:
These equations can be used to solve various problems, you just have to apply equations of motion in projectile motion by considering its independent rectilinear motion along x-axis and y-axis.
Apply equations of motion separately in these two motions:
Where, u = initial velocity, v = final velocity, s = displacement, a = acceleration and t = time.
Time of flight is the total time taken to complete the projectile motion, it will be double the time taken to reach the maximum height. Hence, calculate time to reach maximum height by using equation
And the horizontal range is the displacement of the projectile in x direction. Since, there is no acceleration in x direction so it can be simply calculated using equation
Complete step by step answer:
Let us consider a ball projected at an angle θ with respect to the horizontal x-axis with the initial velocity u as shown below:

In a Projectile Motion, there are always two simultaneous independent rectilinear motions happening:
1. Along the x-axis: along x-axis velocity is uniform which is responsible for the horizontal (forward) motion of the particle.
2. Along y-axis: The particle is moving with a uniform acceleration but varying velocity which is responsible for the vertical (downwards) motion of the particle.
The x components and y components of the initial velocity can be expressed as:
Accelerations in the horizontal and vertical motions of the particle moving in projectile can be expressed as:
There is no acceleration in the horizontal motion of the particle moving in projectile.
The acceleration in the vertical motion of the particle in projectile is just due to acceleration due to gravity:
The velocity of the particle moving in projectile at any point in path of projectile can be given as:
The horizontal velocity remains constant,
But the vertical velocity varies linearly, because the acceleration is constant. At any time, t, the velocity is:
Now, finding:
1. Time of flight:
As we know velocity at max height, v = 0
Using:
Ball will take the same time to return to the ground.
Time of flight :
Horizontal Range = Horizontal Velocity x Flight Time
Hence, proved.
Note:
In projectile motion at any time t, the displacement components are given by:
These equations can be used to solve various problems, you just have to apply equations of motion in projectile motion by considering its independent rectilinear motion along x-axis and y-axis.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




