
What is radial distribution function? Draw this function for the
Answer
456.9k+ views
1 likes
Hint: Radial distribution function tells us about the regions having high and low electron density and we can easily draw the probability curves for the orbitals of hydrogen atom if we know the number of regions of high probability i.e. n and zero probability i.e. l. For s-orbitals; n=n and
L=
Complete answer:
First of all, let’s discuss what a radial distribution function is. By the radial distribution function, we mean the probability of finding the electron within a small radial space around the nucleus.
Radial distribution function is also known as the radial probability distribution .
It depends upon the probability density and the volume of the shell.
The mathematical expression for the radial distribution function is as;
Probability=
Here, gives the probability density for an electron in a given volume , r is the radius and dr is the thickness of the shell.
We can draw the radial distribution curves for the given orbitals if we know the regions of high probability of electrons and zero probability of electrons.
For s-orbitals;
Number of regions of high probability is represented by n and is =
Here, n represents the principal quantum number i.e. the shell to which the electron belongs.
Number of regions zero probability i.e. node is represented by l and is =
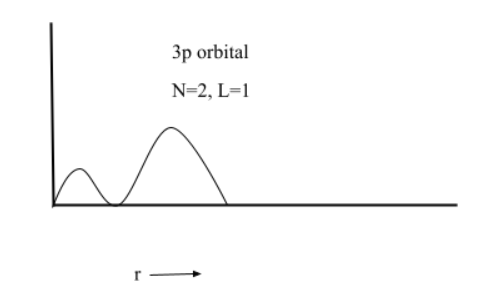
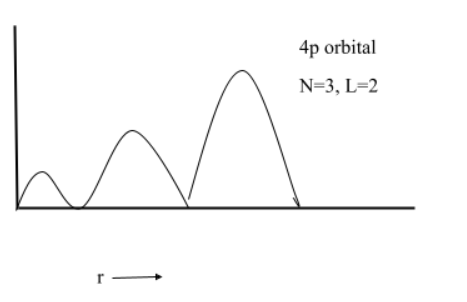
For p-orbitals;
Number of regions of high probability is represented by n and is =
Here, n represents the principal quantum number i.e. the shell to which the electron belongs.
Number of regions zero probability i.e. node is represented by l and is =
Now, the radial probability curves for the
(1)
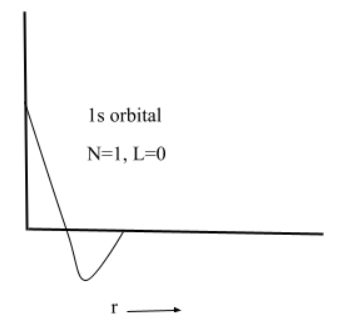
Here, n represents the number of regions of high probability and l represents the number of nodes i.e. the region where probability of finding the electrons is zero.
(2)
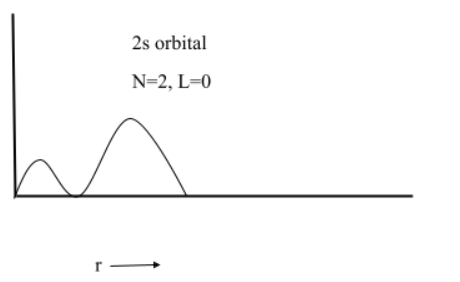
(3)
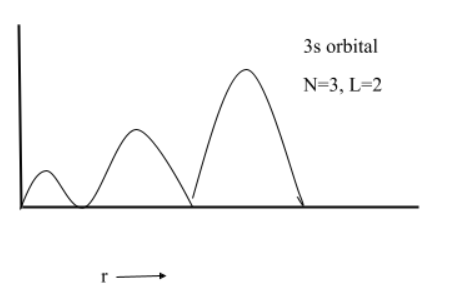
(4)
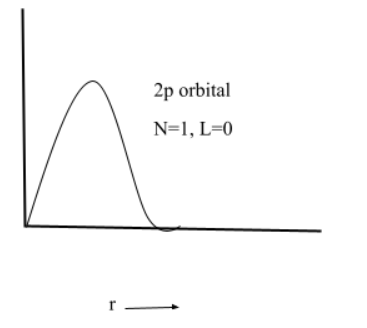
(5)
(6)
Note:
The peak of the curve gives the distance from the nucleus where the probability of finding the electrons is maximum and this distance is called the distance of maximum probability or the radius of maximum probability.
L=
Complete answer:
First of all, let’s discuss what a radial distribution function is. By the radial distribution function, we mean the probability of finding the electron within a small radial space around the nucleus.
Radial distribution function is also known as the radial probability distribution .
It depends upon the probability density and the volume of the shell.
The mathematical expression for the radial distribution function is as;
Probability=
Here, gives the probability density for an electron in a given volume , r is the radius and dr is the thickness of the shell.
We can draw the radial distribution curves for the given orbitals if we know the regions of high probability of electrons and zero probability of electrons.
For s-orbitals;
Number of regions of high probability is represented by n and is =
Here, n represents the principal quantum number i.e. the shell to which the electron belongs.
Number of regions zero probability i.e. node is represented by l and is =
For p-orbitals;
Number of regions of high probability is represented by n and is =
Here, n represents the principal quantum number i.e. the shell to which the electron belongs.
Number of regions zero probability i.e. node is represented by l and is =
Now, the radial probability curves for the
(1)

Here, n represents the number of regions of high probability and l represents the number of nodes i.e. the region where probability of finding the electrons is zero.
(2)

(3)

(4)

(5)

(6)

Note:
The peak of the curve gives the distance from the nucleus where the probability of finding the electrons is maximum and this distance is called the distance of maximum probability or the radius of maximum probability.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




