Question

Ratio of CSA & TSA of cylinder is 1:2, find the volume, if TSA 616 $cm^{2}$.

Answer 1

Hint: In this question it is given that the ratio of CSA(Curved Surface Area) TSA (Total Surface Area) of cylinder is 1:2, we have to find the volume of the cylinder if TSA 616 $cm^{2}$. So to find the solution we need to first use the TSA that is given 616 $cm^{2}$ and the ratio of CSA and TSA. So from the above we will get two equations, which will help us to find the volume of cylinder i.e, volume=$\pi r^{2}h$. To understand it in a better way we have to draw the diagram-

Complete step by step answer:
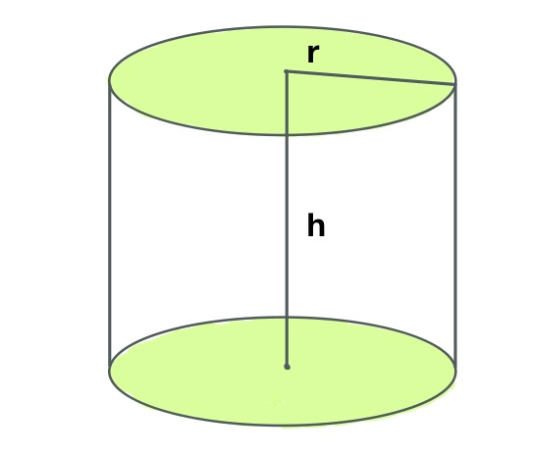
For this problem let us consider the radius of the cylinder be r and the height be h.
Then as we know that CSA=$2\pi rh$ …………(1)
And the TSA=$2\pi r\left( h+r\right) $.................(2)
So in this question it is given that,
TSA=616 $cm^{2}$ ………………(3)
Also it is given, the ratio of CSA & TSA of cylinder is 1:2,
$\therefore$ CSA : TSA=1:2.
$$\Rightarrow \dfrac{CSA}{TSA} =\frac{1}{2}$$
$$\Rightarrow \dfrac{CSA}{616} =\frac{1}{2}$$ [using equation (1)]
$$\Rightarrow CSA=\dfrac{1}{2} \times 616$$ [by cross multiplication]
$$\Rightarrow CSA=308$$
$$\Rightarrow 2\pi rh=308$$ $cm^{2}$........(4) [ since, CSA=$2\pi rh$]
Now from equation (2) and (3), we can write,
$$2\pi r\left( h+r\right) $$=616
$$\Rightarrow 2\pi rh+2\pi r^{2}=616$$
$$\Rightarrow 308+2\pi r^{2}=616$$ [since,$2\pi rh=308$]
$$\Rightarrow 2\pi r^{2}=616-308$$
$$\Rightarrow 2\pi r^{2}=308$$
$$\Rightarrow r^{2}=\dfrac{308}{2\pi }$$
$$\Rightarrow r^{2}=\dfrac{308\times 7}{2\times 22}$$ [ since, $\pi =\dfrac{22}{7}$]
$$\Rightarrow r^{2}=\dfrac{154\times 7}{22}$$
$$\Rightarrow r^{2}=49$$
$$\Rightarrow r^{2}=7^{2}$$
$$\Rightarrow r=7$$
So we get the radius r=7 cm.
Now from equation (4),
$2\pi rh=308$
$$\Rightarrow 2\times \dfrac{22}{7} \times 7\times h=308$$
$$\Rightarrow 44h=308$$
$$\Rightarrow h=\dfrac{308}{44}$$
$$\Rightarrow h=7$$
So we get the height of the cylinder h=7 cm.

Then the volume of cylinder
V =$$\pi r^{2}h$$
=$$\dfrac{22}{7} \times 7^{2}\times 7\ cm^{3}$$
=$$22\times 7^{2}\ cm^{3}$$
=1078 $cm^{3}$.
Therefore the volume of the cylinder is 1078 $cm^{3}$.

Note: While solving this type of problem you need to know that every quantity of cylinder is dependent upon its radius and height so to find volume you have to first find the radius and height. Also always remember that the TSA(Total Surface Area) includes the CSA(Curved Surface Area) and the area of the upper and lower circular face of the cylinder.