
What is the relation between sides opposite to equal angles?
(a) Equal
(b) Unequal
(c) May or may not be equal
(d) None of these
Answer
507.3k+ views
Hint: Construct an angle bisector from the vertex where the sides opposite to equal angles intersect and then using the properties of congruence of triangle, determine the relation between these two sides.
Complete step-by-step answer:
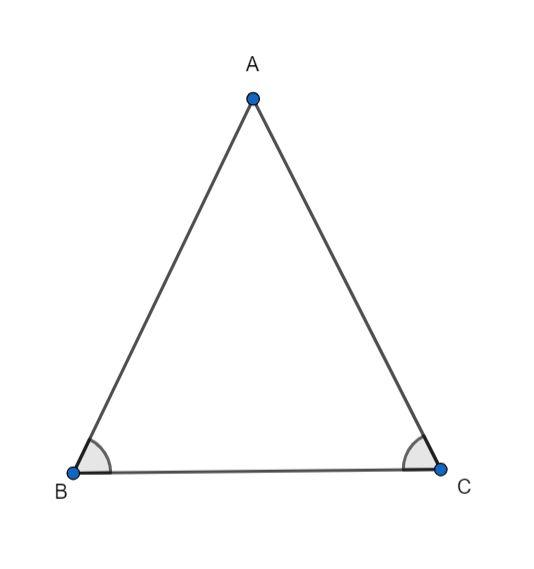
Consider triangle ABC, where angle B and angle C are equal. We need to determine the relation between sides AC and AB which are opposite to the angles B and C respectively.
It is given that angle B and angle C are equal, hence, we have:
\[\angle ABC = \angle ACB............(1)\]
We can determine the relation between sides AB and AC by constructing an angle bisector from vertex A and considering the resulting two triangles.
We draw an angle bisector AD from vertex A to intersect BC at D. Now, we have two triangles, triangle ADB and triangle ADC.
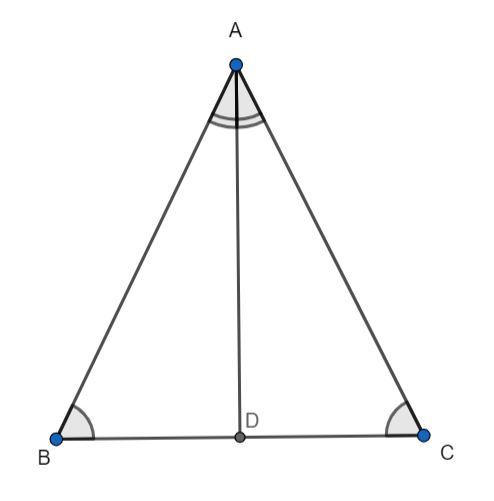
Let us check the triangles ADC and ADB for congruency.
We know that angle ABC is equal to angle ABD and angle ACB is equal to angle ACD.
\[\angle ABD = \angle ABC...........(2)\]
\[\angle ACD = \angle ACB...........(3)\]
Using equation (2) and (3) in equation (1), we have:
\[\angle ABD = \angle ACD...........(4)\]
Since, AD is the angular bisector, it divides angle A into equal angles as follows:
\[\angle BAD = \angle CAD...........(5)\]
The side AD is common to both the triangles. Hence, we have:
\[AD = AD...........(6)\]
From equations (4), (5) and (6), we observe that the two triangles are congruent by AAS (Angle-Angle-Side) criterion.
\[\Delta ABD = \Delta ACD {\text{ (AAS - criterion)}}\]
We know that the ratio of the corresponding sides of congruent triangles are equal.
\[\dfrac{{AB}}{{AC}} = \dfrac{{BD}}{{CD}} = \dfrac{{AD}}{{AD}}\]
Considering the first and the last term, we have:
\[\dfrac{{AB}}{{AC}} = \dfrac{{AD}}{{AD}} = 1\]
\[AB = AC\]
Hence, the two sides are equal.
Hence, the correct answer is option (a).
Note: You may make a mistake if you try to construct a median from the vertex joining the sides opposite to equal angles and try to prove congruence between resulting triangles by SSA criterion, which does not exist for proving congruence between two triangles.
Complete step-by-step answer:

Consider triangle ABC, where angle B and angle C are equal. We need to determine the relation between sides AC and AB which are opposite to the angles B and C respectively.
It is given that angle B and angle C are equal, hence, we have:
\[\angle ABC = \angle ACB............(1)\]
We can determine the relation between sides AB and AC by constructing an angle bisector from vertex A and considering the resulting two triangles.
We draw an angle bisector AD from vertex A to intersect BC at D. Now, we have two triangles, triangle ADB and triangle ADC.

Let us check the triangles ADC and ADB for congruency.
We know that angle ABC is equal to angle ABD and angle ACB is equal to angle ACD.
\[\angle ABD = \angle ABC...........(2)\]
\[\angle ACD = \angle ACB...........(3)\]
Using equation (2) and (3) in equation (1), we have:
\[\angle ABD = \angle ACD...........(4)\]
Since, AD is the angular bisector, it divides angle A into equal angles as follows:
\[\angle BAD = \angle CAD...........(5)\]
The side AD is common to both the triangles. Hence, we have:
\[AD = AD...........(6)\]
From equations (4), (5) and (6), we observe that the two triangles are congruent by AAS (Angle-Angle-Side) criterion.
\[\Delta ABD = \Delta ACD {\text{ (AAS - criterion)}}\]
We know that the ratio of the corresponding sides of congruent triangles are equal.
\[\dfrac{{AB}}{{AC}} = \dfrac{{BD}}{{CD}} = \dfrac{{AD}}{{AD}}\]
Considering the first and the last term, we have:
\[\dfrac{{AB}}{{AC}} = \dfrac{{AD}}{{AD}} = 1\]
\[AB = AC\]
Hence, the two sides are equal.
Hence, the correct answer is option (a).
Note: You may make a mistake if you try to construct a median from the vertex joining the sides opposite to equal angles and try to prove congruence between resulting triangles by SSA criterion, which does not exist for proving congruence between two triangles.
Recently Updated Pages
One difference between a Formal Letter and an informal class null english null

Can anyone list 10 advantages and disadvantages of friction

What are the Components of Financial System?

How do you arrange NH4 + BF3 H2O C2H2 in increasing class 11 chemistry CBSE

Is H mCT and q mCT the same thing If so which is more class 11 chemistry CBSE

What are the possible quantum number for the last outermost class 11 chemistry CBSE

Trending doubts
10 examples of friction in our daily life

The correct order of melting point of 14th group elements class 11 chemistry CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What is the specific heat capacity of ice water and class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE




