
Represent
Answer
435.5k+ views
Hint: Use the fact that if two sides of a right-angled triangle are
Complete step-by-step answer:
Consider a right-angled triangle with side length as 1,1 as shown below
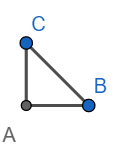
Hence
Draw CD perpendicular BC and CD = 1 unit as shown below.
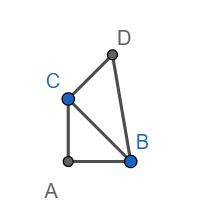
Hence
Draw CE perpendicular to BD, CE = 1 unit.
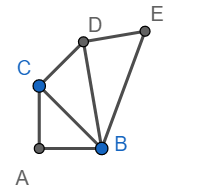
Hence
Draw EF perpendicular to BE, EF = 1 unit as shown below
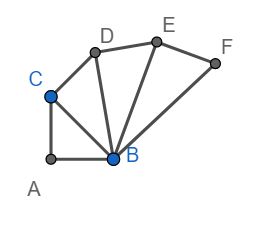
Hence
Draw FG perpendicular BF and FG = 1 unit as shown below.
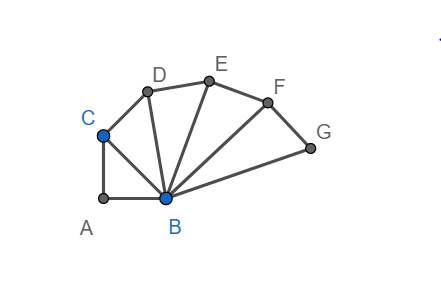
Hence
Draw GH perpendicular BG and GH = 1 unit, as shown below.
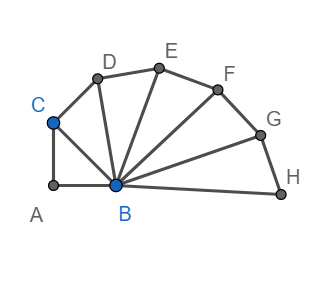
Hence
With O as centre and radius BH, mark draw an arc and let it intersect the positive x-axis at M. M represents
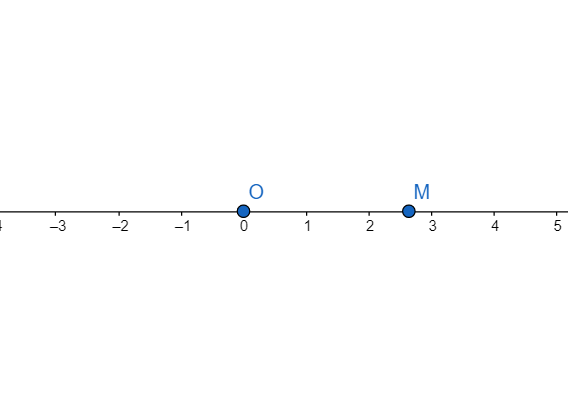
Hence
Note: Alternative method: Best Method:
Draw OX = 7 units.
Extend OX to P such that XP = 1 unit.
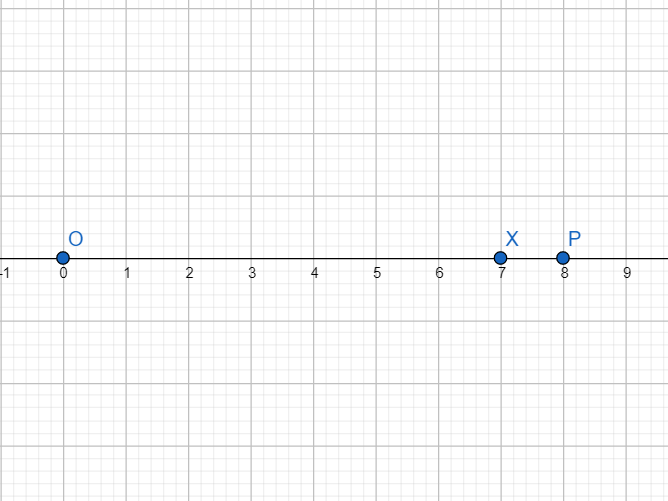
Now locate the midpoint of OP by drawing perpendicular bisector of OP. Let it intersect OP at A as shown below.
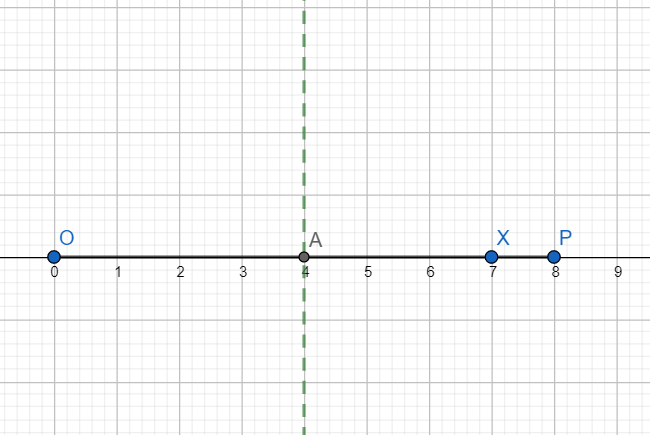
With A as centre and radius OP draw a semicircle as shown below
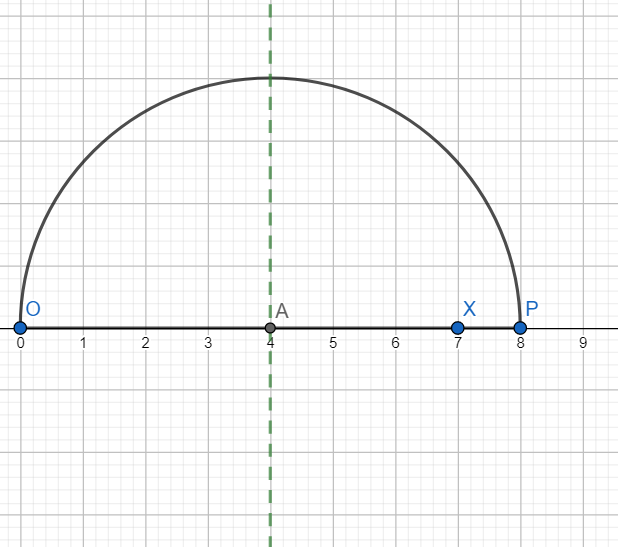
Now draw a line parallel to the perpendicular bisector through X and let it intersect the semicircle at B as shown below.
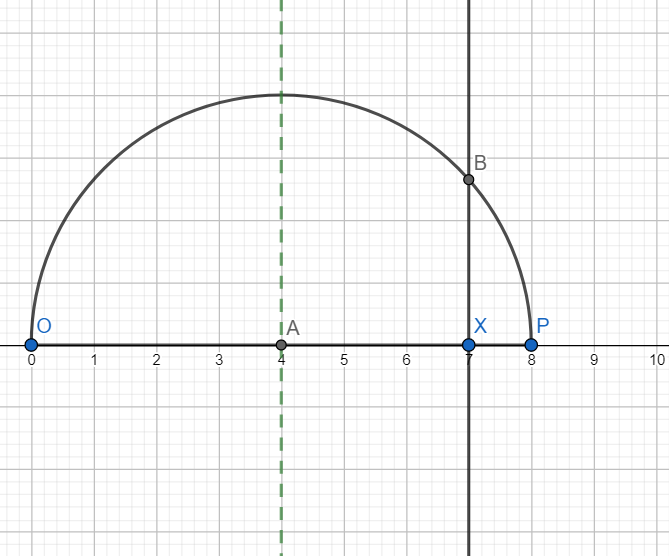
Hence
Now extend compass to be of radius XB draw an arc from O and let it intersect OP at C.
C represents
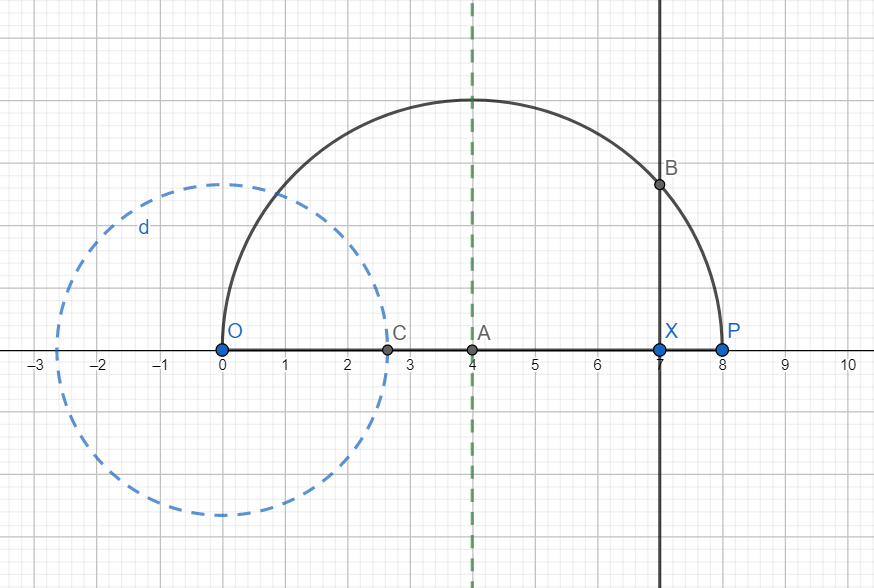
Complete step-by-step answer:
Consider a right-angled triangle with side length as 1,1 as shown below

Hence
Draw CD perpendicular BC and CD = 1 unit as shown below.

Hence
Draw CE perpendicular to BD, CE = 1 unit.

Hence
Draw EF perpendicular to BE, EF = 1 unit as shown below

Hence
Draw FG perpendicular BF and FG = 1 unit as shown below.

Hence
Draw GH perpendicular BG and GH = 1 unit, as shown below.

Hence
With O as centre and radius BH, mark draw an arc and let it intersect the positive x-axis at M. M represents

Hence
Note: Alternative method: Best Method:
Draw OX = 7 units.
Extend OX to P such that XP = 1 unit.

Now locate the midpoint of OP by drawing perpendicular bisector of OP. Let it intersect OP at A as shown below.

With A as centre and radius OP draw a semicircle as shown below

Now draw a line parallel to the perpendicular bisector through X and let it intersect the semicircle at B as shown below.

Hence
Now extend compass to be of radius XB draw an arc from O and let it intersect OP at C.
C represents

Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




