
Round off to nearest thousands. The given number is
Answer
477.3k+ views
Hint: Here we will either round up or round down the number to its nearest thousands as per the face value of the digit in the hundreds place. On doing some simplification we get the required answer.
Formula used: Suppose a number is in the form of thousands.
Let say, the number is
So, if we want to make this number to its nearest thousands, then we will follow the following rules:
So, the digit in hundreds place will be
And, other digits will become zero,
So, the digit in hundreds places will be
And, other digits will become zero,
Complete step-by-step solution:
The given number is
Now, the digit in hundreds places is
So, as per the above rules:
We have to add
So, the digit in thousand’s place will become
So, after rounding off, the required nearest thousands of
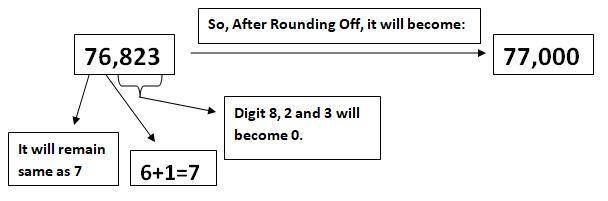
Option C is the correct answer.
Note: An alternate process can be used to solve this kind of problem.
We can draw this number in a line graph and then we can check whether the number is closest to the latter half or the earlier half.
So, we will draw a range of
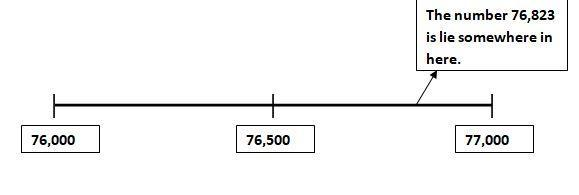
So, if we look at it closely, we can say that
So, rounding off to the nearest thousands means finding the number which is nearest to multiple of its thousand’s place.
So, we can say that
Formula used: Suppose a number is in the form of thousands.
Let say, the number is
So, if we want to make this number to its nearest thousands, then we will follow the following rules:
So, the digit in hundreds place will be
And, other digits will become zero,
So, the digit in hundreds places will be
And, other digits will become zero,
Complete step-by-step solution:
The given number is
Now, the digit in hundreds places is
So, as per the above rules:
We have to add
So, the digit in thousand’s place will become
So, after rounding off, the required nearest thousands of

Option C is the correct answer.
Note: An alternate process can be used to solve this kind of problem.
We can draw this number in a line graph and then we can check whether the number is closest to the latter half or the earlier half.
So, we will draw a range of

So, if we look at it closely, we can say that
So, rounding off to the nearest thousands means finding the number which is nearest to multiple of its thousand’s place.
So, we can say that
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

What is the feminine gender of a stag class 8 english CBSE

Write five sentences about Earth class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE




