
Savitri had to make a model of a cylindrical kaleidoscope for her science project. She wanted to use the chart paper to make the curved surface of the kaleidoscope. What would be the area of chart paper required by her, if she wanted to make a kaleidoscope of length 25cm with a 3.5cm radius? You may take
(A). 540
(B). 520
(C). 550
(D). 560
Answer
519.9k+ views
Hint: Use the fact that a kaleidoscope is a cylindrical device with each of its circular ends fitted with reflecting surfaces like mirrors. Also, use the formula of the curved surface of a cylinder.
Complete step-by-step solution -
Let us start by drawing a representative figure for better visualisation.
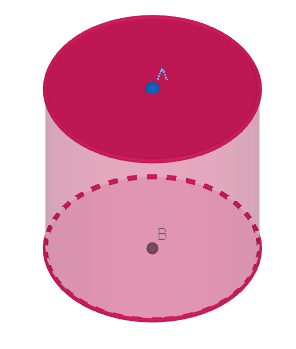
Before we start with the solution, let us discuss about kaleidoscope. A kaleidoscope is an optical instrument generally used to show beautiful designs formed by the repetition of the same artistic elements in a symmetrical form. It is cylindrical in shape, hollow from inside, and contains two reflecting surfaces at its two ends.
Now according to the question, we need to find the curved surface area of the cylindrical kaleidoscope as the area of chart paper needed is equal to the curved surface area of the cylinder. We know that the curved surface area of a cylinder is
Now, as given in the question, we will put the value of
The curved surface area of cylinder =
Therefore, the area of the chart paper required is
Note: Make sure to convert all the dimensions to a standardized system of units; this decreases the chance of errors. It would also help if you remembered all the basic formulas for surface area and volume of the general 3-D shapes like the cone, cube, cylinder, etc.
Complete step-by-step solution -
Let us start by drawing a representative figure for better visualisation.

Before we start with the solution, let us discuss about kaleidoscope. A kaleidoscope is an optical instrument generally used to show beautiful designs formed by the repetition of the same artistic elements in a symmetrical form. It is cylindrical in shape, hollow from inside, and contains two reflecting surfaces at its two ends.
Now according to the question, we need to find the curved surface area of the cylindrical kaleidoscope as the area of chart paper needed is equal to the curved surface area of the cylinder. We know that the curved surface area of a cylinder is
Now, as given in the question, we will put the value of
The curved surface area of cylinder =
Therefore, the area of the chart paper required is
Note: Make sure to convert all the dimensions to a standardized system of units; this decreases the chance of errors. It would also help if you remembered all the basic formulas for surface area and volume of the general 3-D shapes like the cone, cube, cylinder, etc.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




