
Select incorrect alternative.
A)
B)
C)
D)
Answer
495.9k+ views
Hint: Find the incorrect value in the above given trigonometric functions. Use these formulas to find the incorrect one
Complete step-by-step answer:
We are given four options and we have to find the incorrect one from them.
(A)
If a triangle has sides 3, 4, 5 then it is definitely a right angled triangle because the square of 5 is 25 which is equal to square of 3 and 4 which is 16, 9 and one angle of the triangle will be 90 as it is a right triangle and other angles will be 35 and 53 (measure using a protractor).
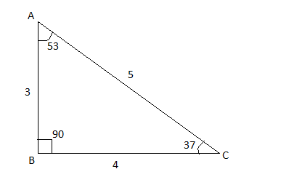
Opposite side of angle 37 is AB and hypotenuse is AC
Therefore, Option A is correct.
(B)
We got that
We know that
So
By Pythagorean trigonometric identity we have
To calculate the value of
Therefore, Option B is also correct.
(C)
We know that tangent function is the ratio of sine function and cosine function.
Therefore
But given that
Therefore Option C is incorrect.
(D)
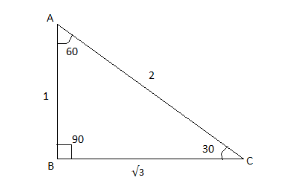
From the triangle, when the sides are 1, √3, 2 then the angles of the triangle are 30, 60, 90.
Adjacent side of angle 30 is BC and the hypotenuse is AC.
Therefore, Option D is also correct.
Options A, B and D are correct and Option C is incorrect.
So, the correct answer is “Option A,B AND D”.
Note: Trigonometry studies relationships between side lengths and angles. In trigonometry, there are three pairs of co-functions. They are sin-cos, tan-cot, cosec-sec. For these co-functions the value of one co-function of x is equal to the value of other cofunction of 90-x.
Complete step-by-step answer:
We are given four options and we have to find the incorrect one from them.
(A)
If a triangle has sides 3, 4, 5 then it is definitely a right angled triangle because the square of 5 is 25 which is equal to square of 3 and 4 which is 16, 9 and one angle of the triangle will be 90 as it is a right triangle and other angles will be 35 and 53 (measure using a protractor).

Opposite side of angle 37 is AB and hypotenuse is AC
Therefore, Option A is correct.
(B)
We got that
We know that
So
By Pythagorean trigonometric identity we have
To calculate the value of
Therefore, Option B is also correct.
(C)
We know that tangent function is the ratio of sine function and cosine function.
Therefore
But given that
Therefore Option C is incorrect.
(D)

From the triangle, when the sides are 1, √3, 2 then the angles of the triangle are 30, 60, 90.
Adjacent side of angle 30 is BC and the hypotenuse is AC.
Therefore, Option D is also correct.
Options A, B and D are correct and Option C is incorrect.
So, the correct answer is “Option A,B AND D”.
Note: Trigonometry studies relationships between side lengths and angles. In trigonometry, there are three pairs of co-functions. They are sin-cos, tan-cot, cosec-sec. For these co-functions the value of one co-function of x is equal to the value of other cofunction of 90-x.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




