
Show that Kinetic energy is always lost in inelastic collisions.
Answer
393k+ views
Hint: In order to solve this question, we should know that inelastic collisions are predefined as those collisions in which momentum remain conserved but kinetic energy lost in form of heat, sound and other forms of energy, here we will understand the perfectly inelastic collision and will figure out the lost in kinetic energy of the system.
Complete step by step answer:
A perfectly inelastic collision is one in which after the collision both the colliding bodies stick together and move with same velocity. Let us suppose two bodies having mass of
Now, let us suppose both bodies collide and stick together and moves with same velocity say v’ then, final mass of the system will be
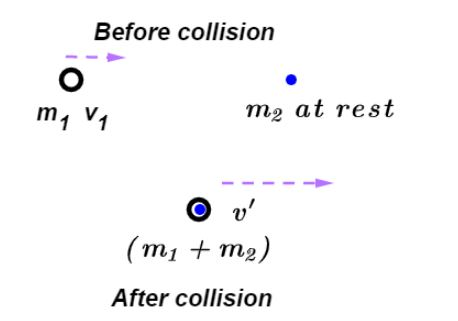
Now, initial momentum will be equal to that of final momentum according to law of conservation of linear momentum so, equation initial and final momentum we get,
Now, let initial kinetic energy of the system be
from equation (i) put the value of
Now, let us find the value of
Hence, there is non-zero value in difference between initial kinetic energy and final kinetic energy which shows that, Kinetic energy is always lost in inelastic collision and this loss has a magnitude of
Note: It should be remembered that, when two bodies stick together their mass will be a combined sum of mass of individual bodies and they will together move with same velocity and this is called a perfectly inelastic collision. while in elastic collision initial kinetic energy and final kinetic energy are always equal.
Complete step by step answer:
A perfectly inelastic collision is one in which after the collision both the colliding bodies stick together and move with same velocity. Let us suppose two bodies having mass of
Now, let us suppose both bodies collide and stick together and moves with same velocity say v’ then, final mass of the system will be

Now, initial momentum will be equal to that of final momentum according to law of conservation of linear momentum so, equation initial and final momentum we get,
Now, let initial kinetic energy of the system be
from equation (i) put the value of
Now, let us find the value of
Hence, there is non-zero value in difference between initial kinetic energy and final kinetic energy which shows that, Kinetic energy is always lost in inelastic collision and this loss has a magnitude of
Note: It should be remembered that, when two bodies stick together their mass will be a combined sum of mass of individual bodies and they will together move with same velocity and this is called a perfectly inelastic collision. while in elastic collision initial kinetic energy and final kinetic energy are always equal.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




