
Sketch the graph for
Answer
477k+ views
Hint:
To sketch the graph of
In a relation R from set A to set B, the set of all first components of order pair belongings to R is called the domain and all the second element of pair is called range.
Complete step by step solution:
We know that the domain of tan. function(tangent function) is the set
here R represents the real number and
The range of the tan function in R. Here R represents the real number. If we restrict the domain of a tangent function to
The graph of a tan function
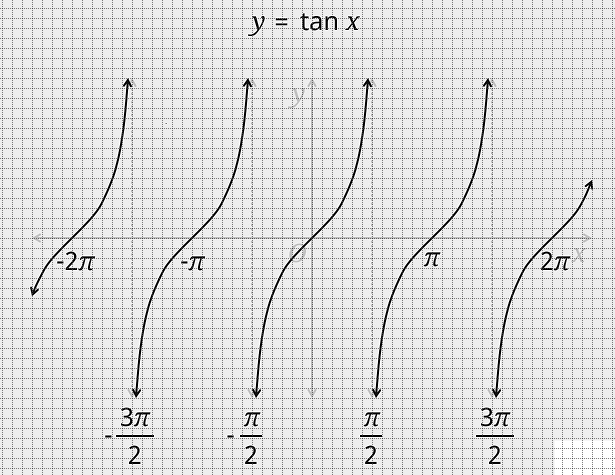
The graph of

As
As we know that
This has been defined for
Thus this graph of y where y is not defined for
Note:
One-one function: A function
Onto function: A function is
Bijective Function: The function which is both one-one and onto is called the bijective function.
To sketch the graph of
In a relation R from set A to set B, the set of all first components of order pair belongings to R is called the domain and all the second element of pair is called range.
Complete step by step solution:
We know that the domain of tan. function(tangent function) is the set
here R represents the real number and
The range of the tan function in R. Here R represents the real number. If we restrict the domain of a tangent function to
The graph of a tan function
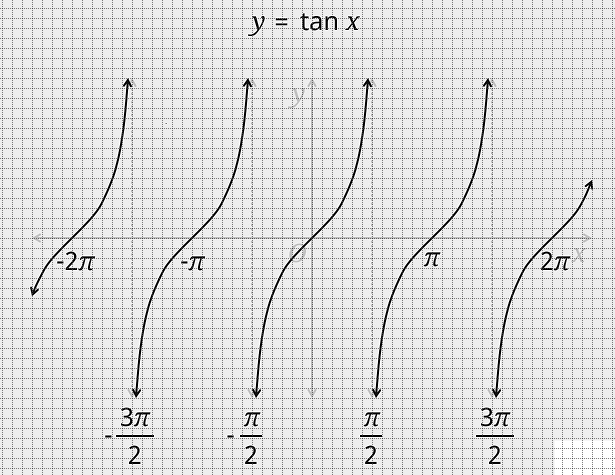
The graph of

As
As we know that
This has been defined for

Thus this graph of y where y is not defined for
Note:
One-one function: A function
Onto function: A function is
Bijective Function: The function which is both one-one and onto is called the bijective function.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




