
Solve:
Answer
516k+ views
Hint: We will first start by arranging the terms in an order such that all the terms with x are one side and the rest terms on another side. Then we will use the principle of inequality to find the range of value of x.
Complete step-by-step answer:
Now, we have been given that
Now, we will move 5x from RHS to LHS by subtracting 5x from both sides and -7 from LHS to RHS by adding +7 on both sides. So, we have,
Now, we know that on multiplying both sides by -1. We have the sign of inequality reversed. So, we have,
Now, dividing both sides by 2 we have,
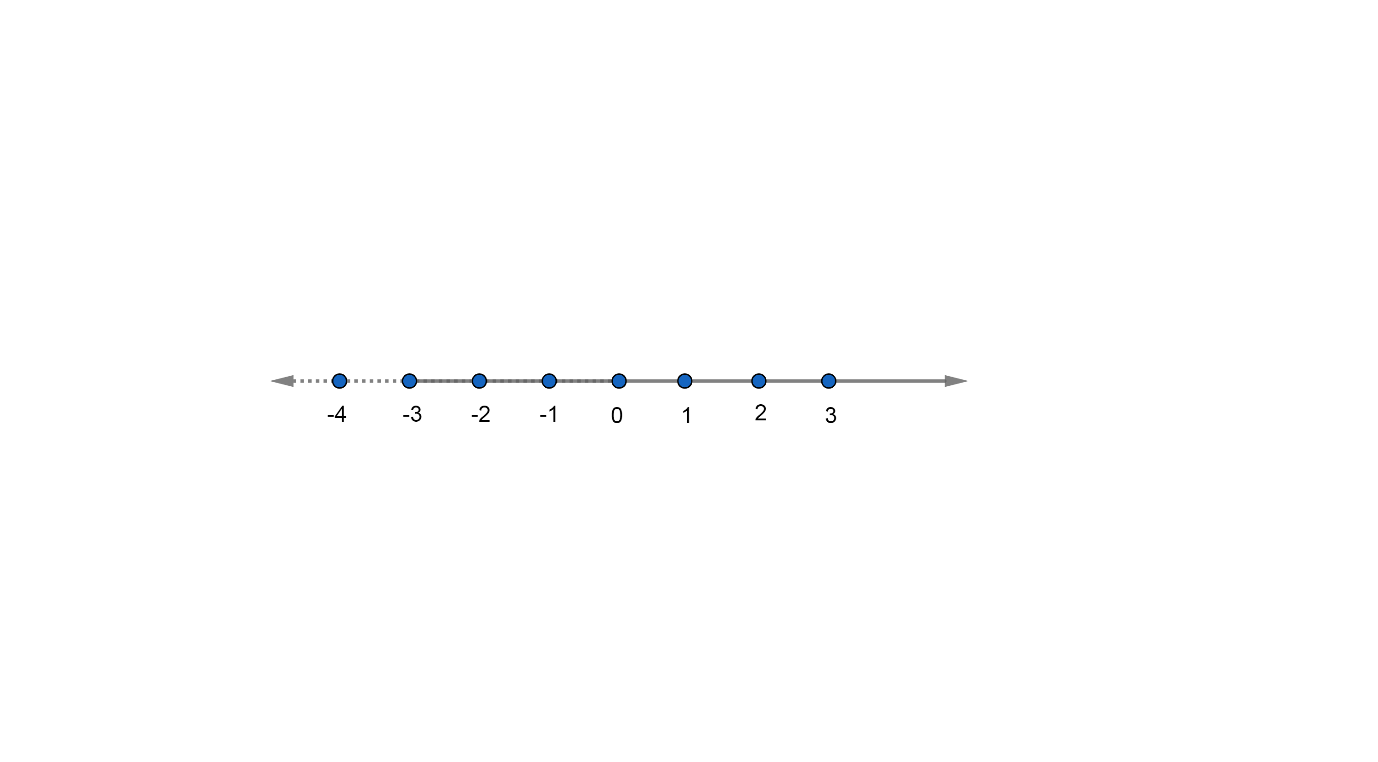
Which can be shown on number line as,
Note: It is important to note that we have changed the sign of inequality on multiplying the both sides by -1. For example, we know that -2 < 3. Now, if we multiply by -1 on both sides with signs of inequality changing. We have +2 < -3 which is not true. Hence, the sign of inequality is changed on multiplying or dividing an inequality by a negative number.
Complete step-by-step answer:
Now, we have been given that
Now, we will move 5x from RHS to LHS by subtracting 5x from both sides and -7 from LHS to RHS by adding +7 on both sides. So, we have,
Now, we know that on multiplying both sides by -1. We have the sign of inequality reversed. So, we have,
Now, dividing both sides by 2 we have,
Which can be shown on number line as,

Note: It is important to note that we have changed the sign of inequality on multiplying the both sides by -1. For example, we know that -2 < 3. Now, if we multiply by -1 on both sides with signs of inequality changing. We have +2 < -3 which is not true. Hence, the sign of inequality is changed on multiplying or dividing an inequality by a negative number.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE




