Question

When sound is produced in an aeroplane moving with a velocity of $200{m}/{s}$ horizontal its echo is heard after $10\sqrt{5}$. If the velocity of sound in air is $300m{s}^{-1}$ the elevation of the aircraft is:
A. 250 m
B. $250\sqrt {5} m$
C. 1250 m
D. 2500 m

Answer 1

Hint: To solve this problem, first divide the time taken to hear the echo by 2 as it is the time taken for the sound to go and come back. Then, use the formula for velocity giving relation between velocity and time. Using this formula, find the distance covered by aeroplane. Similarly, find the distance covered by sound. Using these values of distances, find the elevation of the aircraft.
Formula used:
$Velocity= \dfrac {Distance}{Time}$

Complete answer:

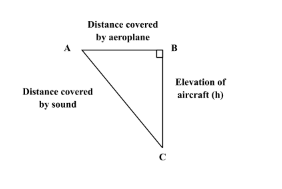
Let h be the elevation of the spacecraft.
Given: Velocity of aeroplane ${v}_{1}$ = $200{m}/{s}$
            Velocity of sound in air ${v}_{2}$ = $300{m}/{s}$
Time for the echo (t) is given as $10\sqrt{5}$. But this is the addition of the times taken by sound to travel forward and the time taken by sound to travel backward. But we need time only for one direction. So, we have to consider time as $\dfrac{t}{2}$.
Velocity is given by,
$Velocity= \dfrac {Distance}{Time}$
Rearranging above equation we get,
$Distance = Velocity \times time$ …(1)
Using equation. (1), distance covered by aeroplane is given by,
$ AB= {v}_{1} \times \dfrac {t}{2}$
Substituting the values in above equation we get,
$AB= 200 \times 5\sqrt{5}$
$\Rightarrow AB= 2236 m$
Similarly, distance covered by sound is given by,
$ AC= {v}_{2} \times \dfrac {t}{2}$
Substituting the values in above equation we get,
$AC= 300 \times 5\sqrt{5}$
$\Rightarrow AB= 3354 m$
From the figure, it can be inferred that,
${AC}^{2} = {AB}^{2} + {BC}^{2}$
Rearranging the above equation we get,
${BC}^{2} = {AC}^{2} - {AB}^{2}$
Substituting the values in above equation we get,
${h}^{2}= {3354}^{2} – {2236}^{2}$
$\Rightarrow {h}^{2}= (11.25 \times {10}^{6}) – (5 \times {10}^{6})$
$\Rightarrow {h}^{2}= 6.25 \times {10}^{6}$
Taking the square root on both the sides we get,
$h = 2.5 \times {10}^{3}$
$\Rightarrow h = 2500m$
Hence, the elevation of the aircraft is 2500 m.

So, the correct answer is “Option D”.

Note:
Students must remember to divide the time given by 2. As we have to consider time taken by sound to travel one-way. Students must also remember that the speed of sound for different mediums is different. So, if there was any other medium, the distance covered by sound would change depending on the density of the medium. Suppose the medium for sound to travel to be water. The sound travels faster in water, so the distance covered by the sound should be greater than what we got.