
Standing waves are produced in
A.
B.
C.
D.
Answer
402.6k+ views
Hint: To answer the question, we will build a simple diagram based on the question. The entire length of the string is
Complete answer:
Before we go into the question, let's have a look at what a standing wave is. The combination of two waves flowing in opposite directions, each with the same amplitude and frequency, is known as a standing wave.
Now, let us come to the question;
The wavelength of a stretched string's fundamental vibrational mode is twice the length of the string.
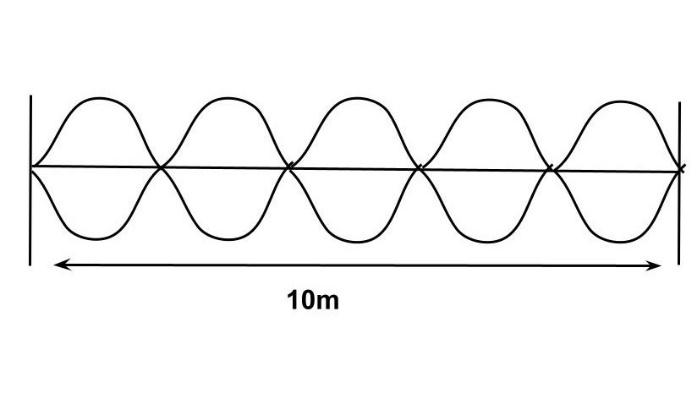
Because the string produces standing waves and vibrates in five parts, it can be shown as
Therefore, from here we will find value of
The wave's velocity,
Hence, the frequency will be
Therefore, the frequency is
The correct option is: (A)
Note:
It's important to note that standing waves don't just appear out of nowhere. They call for energy to be delivered into a system at a specific frequency. That is, when a system's driving frequency is identical to its natural frequency. Resonance is the term for this situation. Standing waves are invariably linked to resonance.
Complete answer:
Before we go into the question, let's have a look at what a standing wave is. The combination of two waves flowing in opposite directions, each with the same amplitude and frequency, is known as a standing wave.
Now, let us come to the question;
The wavelength of a stretched string's fundamental vibrational mode is twice the length of the string.

Because the string produces standing waves and vibrates in five parts, it can be shown as
Therefore, from here we will find value of
The wave's velocity,
Hence, the frequency will be
Therefore, the frequency is
The correct option is: (A)
Note:
It's important to note that standing waves don't just appear out of nowhere. They call for energy to be delivered into a system at a specific frequency. That is, when a system's driving frequency is identical to its natural frequency. Resonance is the term for this situation. Standing waves are invariably linked to resonance.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




