
State and prove the Pythagoras theorem.
Answer
511.5k+ views
37 likes
Hint: Draw a perpendicular on AC from B and use angle-angle similarity to prove the theorem.
Complete step-by-step answer:
Pythagoras theorem states that “ In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.
The sides of the right-angled triangle are called base, perpendicular and hypotenuse .
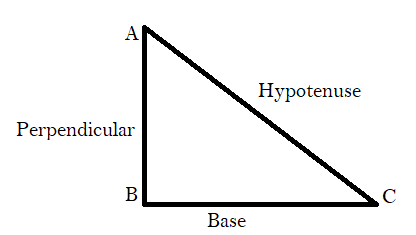
According to Pythagoras theorem ,
Proof:
Given, a triangle ABC in which
Construction: Draw a perpendicular BD on AC i.e. BD
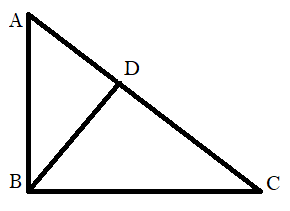
In
Therefore
So,
In
Therefore
So,
Adding equation (1) and (2) , we get
Hence, proved.
Note: In a right angled triangle , hypotenuse is the longest side of the triangle and is opposite to the right angle i.e.
Complete step-by-step answer:
Pythagoras theorem states that “ In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.
The sides of the right-angled triangle are called base, perpendicular and hypotenuse .

According to Pythagoras theorem ,
Proof:
Given, a triangle ABC in which
Construction: Draw a perpendicular BD on AC i.e. BD

In
Therefore
So,
In
Therefore
So,
Adding equation (1) and (2) , we get
Hence, proved.
Note: In a right angled triangle , hypotenuse is the longest side of the triangle and is opposite to the right angle i.e.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What is the past participle of wear Is it worn or class 10 english CBSE

What is the full form of POSCO class 10 social science CBSE

How fast is 60 miles per hour in kilometres per ho class 10 maths CBSE

Choose the feminine form of the given noun Fox AFoxess class 10 english CBSE




