Question

State Rolle’s theorem.

Answer 1

Hint: Rolle’s theorem is a special case of Mean value theorem. So, we will discuss Mean value theorem, then we will state Rolle's theorem with the help of a suitable proof.

Complete step-by-step answer:
It is given in the question to state Rolle’s theorem. So, basically Rolle’s theorem is a special case of Mean value theorem. It can also be called a mean value theorem (MVT). So, (MVT) stated that ‘if $f(x)$ is a function in an interval of $(a,b)$ and $f(x)$ can be differentiable on $(a,b)$ then we have at least one value $c$ in the interval $(a,b)$. Where, $a < c < b$.
${{f}^{1}}(c)=\dfrac{f(b)-f(a)}{b-a}$.
Whereas, Rolle’s theorem is a special case of MVT. Where,
$f(a)=f(b)$. So, here we have ${{f}^{1}}(c)=0$.
${{f}^{1}}(c)$ Rolle’s theorem states that if a function $f$ is continuous on the closed interval $[a,b]$ and differentiable on the open interval $(a,b)$ such that $f(a)=f(b)$. Then ${{f}^{1}}(c)=0$ for some values of $c$ with $a\le c\le b$.

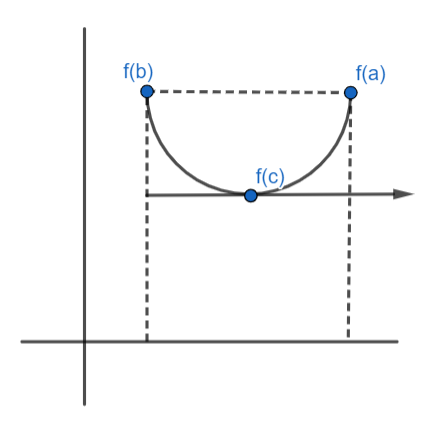
Here in this diagram we have function $f(b)=f(a)$ and ${{f}^{1}}(c)$ is parallel to x-axis which means ${{f}^{1}}(c)=0$. So, $f$ is continuous on $[a,b]$. Therefore, it assumes absolute maximum and minimum values on $[a,b]$.

Note: Rolle’s theorem is important because it gives a necessary condition for critical points; it is used in the proof of the Intermediate value theorem. In fact, IVT is similar to Rolle’s theorem. Rolle’s theorem states that ‘If a function $f(x)$ is defined in the closed interval $[a,b]$ in such a way that it satisfies the following conditions –
1. The function $f(x)$ is continuous in $[a,b]$.
2. $f(x)$ is differentiable in $(a,b)$.
3. $f(a)=f(b)$.
Then there exists at least one value of $x$ such that ${{f}^{1}}(c)=0$’.