
State the law of parallelogram of vector addition and find the magnitude and direction of the resultant of vectors P and Q inclined at an angle
Answer
504.9k+ views
Hint: At First sketch out how your vectors and its resultant would look on a parallelogram. Then label the angle between the two vectors as
Formula Used:
Parallelogram law of vector addition for the resultant of two vectors:
Magnitude of the resultant vector
Direction of the resultant
Complete step-by-step solution:
Let us begin with the parallelogram law of vector addition.
The law states that if two vector quantities are represented by two adjacent sides of a parallelogram then the resultant of these two vectors will be the diagonal of the parallelogram.
Let us try and understand how this works:
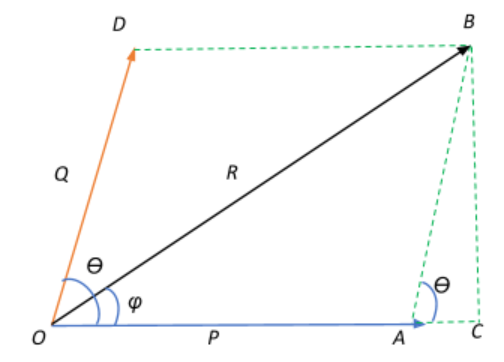
Let the two vectors
Let the angle between the two vectors be
According to our definition of the parallelogram law of vector addition, the diagonal of the parallelogram OB represents the resultant of
The sides
Consider the right angle triangle OBC. From the Pythagorean theorem:
Consider the triangle ABC:
Also
Substituting the AC and BC expressions in the
Therefore, the magnitude of the resultant
Now let us find the direction of the resultant:
From triangle ABC
Therefore, the direction of the resultant with respect to
We have thus obtained the magnitude and direction of the resultant
Now, when
Thus, in this case, the magnitude of the resultant vector will be the sum of the magnitudes of the adjacent vectors and the the resultant lies in the direction of
And finally, when
Note: It is important to notice that the direction of the resultant that we have obtained in our above derivation is the angle that the resultant makes with only the vector
Formula Used:
Parallelogram law of vector addition for the resultant of two vectors:
Magnitude of the resultant vector
Direction of the resultant
Complete step-by-step solution:
Let us begin with the parallelogram law of vector addition.
The law states that if two vector quantities are represented by two adjacent sides of a parallelogram then the resultant of these two vectors will be the diagonal of the parallelogram.
Let us try and understand how this works:

Let the two vectors
Let the angle between the two vectors be
According to our definition of the parallelogram law of vector addition, the diagonal of the parallelogram OB represents the resultant of
The sides
Consider the right angle triangle OBC. From the Pythagorean theorem:
Consider the triangle ABC:
Also
Substituting the AC and BC expressions in the
Therefore, the magnitude of the resultant
Now let us find the direction of the resultant:
From triangle ABC
Therefore, the direction of the resultant with respect to
We have thus obtained the magnitude and direction of the resultant
Now, when
Thus, in this case, the magnitude of the resultant vector will be the sum of the magnitudes of the adjacent vectors and the the resultant lies in the direction of
And finally, when
Note: It is important to notice that the direction of the resultant that we have obtained in our above derivation is the angle that the resultant makes with only the vector
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




