Question

State triangle law of vector addition. Give analytical treatment to find the magnitude and direction of a resultant vector by using this law.

Answer 1

Hint: Triangle law of addition is the sum of two vectors but in a unique way. Triangle law of vector addition states that when two vectors are represented as two sides of the triangle with the order of magnitude and direction, then the third side of the triangle represents the magnitude and direction of the resultant vector. You can use this law in abuse as well as obtuse angles.

Complete step by step answer:
Two vectors A and B are said to be equal if and only if they have the same direction and magnitude.
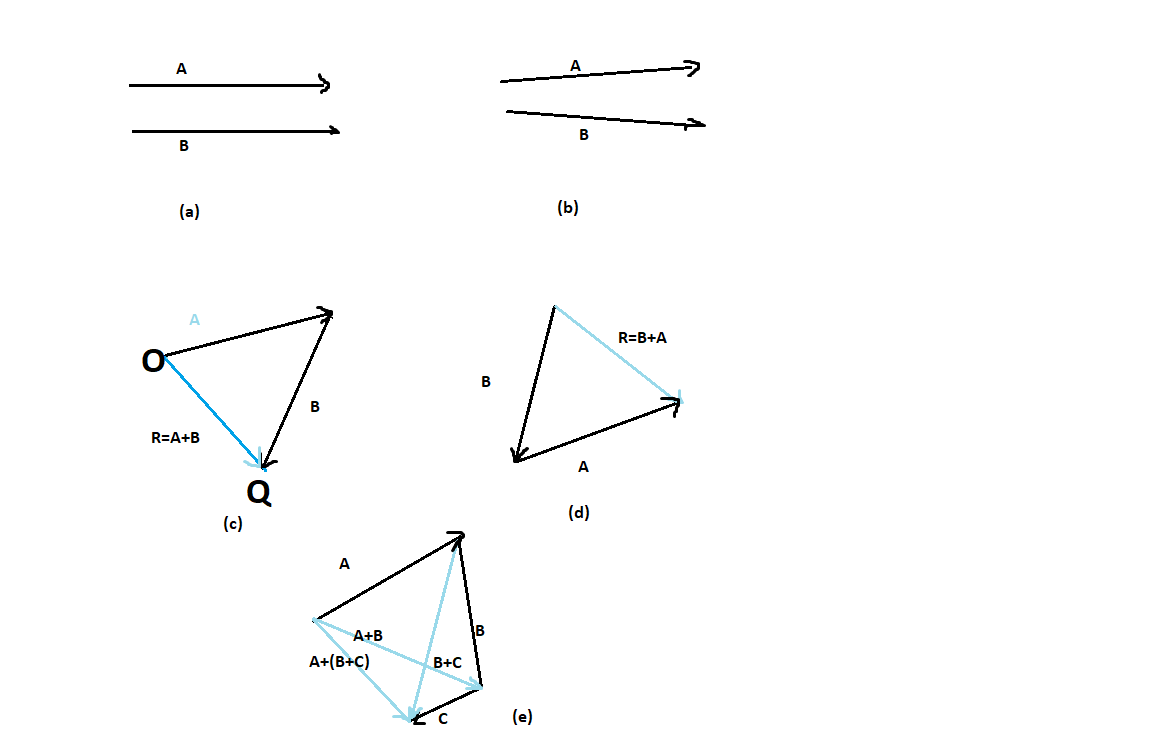
As you can see in figure. Figure (a) is equal but (b) is not equal.
Let’s consider two vectors A and B. They have the same magnitude but they are not in the same direction.
Now to find the sum of A and B i.e.$$A+B$$. We place vector B so that its tail is at the head of vector A as shown in (c). Then joining the tail of A to the head of B. OQ represents a vector R, that is, the sum of vectors A and B. Since, in this procedure of vector addition, vectors are arranged head to tail, this graphical method is called the head to tail method. The two vectors and their resultant form three Side. If we find the resultant of B+A as shown in figure (d), the same vector R is obtained. Thus vector addition is commutative which is given by
$$A+B=B+A$$
The addition of vectors also obeys the associative law which is shown in figures (e). The result of adding vectors A and B first and then adding vector C is same as the result of adding B and C first and then adding vector A:
$$(A+B)+C=A+(B+C)$$of a triangle, so this method is also known as the triangle method of vector addition.
Diagram:

Note: Vector quantity is the quantity which has both magnitude and direction. Along with triangle law of addition, subtraction and multiplication of vectors are also possible. The vector addition must follow commutative and associative law. If two vectors represented as sides are parallel to each other or if they have an angle of 180 degree then you cannot use a triangle of vector addition.