
Sunlight of intensity
Answer
463.8k+ views
1 likes
Hint: This is a question of ray optics, so first draw a ray diagram with the given information and check where the rays after emerging from the lens would meet. In this question we will also have to use the properties of similar triangles and use the formula for intensity to find the required answer.
Complete step by step solution:
Given, intensity of sunlight,
Focal length of convex lens,
Distance at which the average intensity has to be found from the other side of the lens,
We draw the ray diagram with the given conditions,
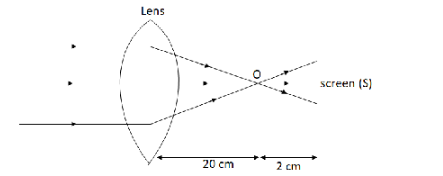
We know, rays from infinite distance or parallel to the optical axis fall on a converging lens, the emerging rays intersect at the focal point on the other side of the lens.
Here, the focal point on the other side is
The distance of the screen from the other side is
Let
Now, let us redraw the figure
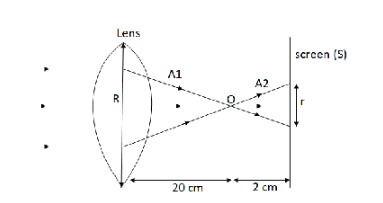
We can observe that the triangles
Putting the values of
Intensity can be defined as energy per unit area, that is
When the rays on sunlight incident on the lens, its intensity will be
where
Putting this value in above equation we get,
The intensity on the screen will be (using the fact that there is no loss in energy so energy will be the same)
where
Dividing equation (iii) by (ii), we get
Putting the values of
Therefore, the average intensity of light at a distance of
Note: Whenever you have a question of ray optics, always draw a ray diagram with the information given in the question. And with the help of the diagram find the required quantity. Also remember rays from infinite distance are considered as parallel rays, this is because as the distance between the source and the lens or mirror increases, the angle between rays from a distance source approaches zero.
Complete step by step solution:
Given, intensity of sunlight,
Focal length of convex lens,
Distance at which the average intensity has to be found from the other side of the lens,
We draw the ray diagram with the given conditions,

We know, rays from infinite distance or parallel to the optical axis fall on a converging lens, the emerging rays intersect at the focal point on the other side of the lens.
Here, the focal point on the other side is
The distance of the screen from the other side is
Let
Now, let us redraw the figure

We can observe that the triangles
Putting the values of
Intensity can be defined as energy per unit area, that is
When the rays on sunlight incident on the lens, its intensity will be
where
Putting this value in above equation we get,
The intensity on the screen will be (using the fact that there is no loss in energy so energy will be the same)
where
Dividing equation (iii) by (ii), we get
Putting the values of
Therefore, the average intensity of light at a distance of
Note: Whenever you have a question of ray optics, always draw a ray diagram with the information given in the question. And with the help of the diagram find the required quantity. Also remember rays from infinite distance are considered as parallel rays, this is because as the distance between the source and the lens or mirror increases, the angle between rays from a distance source approaches zero.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹35,000 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




