Answer
492k+ views
Hint: We will use the property that the tangent from some point to the circle has some length. The length of PA is equal to the length of PB because the two tangents from a point to the circle are equal.
Complete step by step answer:

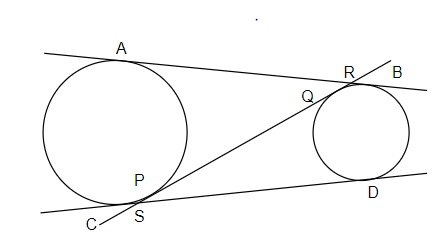
it is given in the question that S1 and S2 are two unequal circles. Also, AB and CD are the direct common tangents to the circles. It is also given that a transverse common tangent PQ cuts AB in R and CD in S, and length of AB=10 then we have to find the length of RS.
We know that the length of two tangents from a point to the circle are of equal length. So, we get $RQ=RB$ and $SC=SP$. Let us assume that $RQ=RB=x$ and $SC=SP=y$. Now, we know that the length of AB is 10 units, so we get length $AR=AB-RB=RP=10-x$. AR and RP are the two tangents from a common point R to the circle S1 thus are of equal length.
As we have $AR=AB-RB=RP=10-x$ and $RQ=x$, so from this we get \[PQ=RP-RQ=10-x-x\] or $PQ=10-2x$. Again the tangents SQ and SD are equal because both are common on point S and circle ${{S}_{2}}$, and also we had assumed that \[SP=y\] and we have already calculated \[PQ=10-2x\]. Therefore, we get \[SQ=SP+PQ=y+10-2x=SD\].
We also know that the length of direct common tangents of two circles are equal thus tangents $AB=CD=10$. Now, we get $CD=CS+SD$ and we have $CD=10,CS=y$ and $SD=10-2x+y$. On putting the value of CD, CS and SD, we get $10=y+10-2x+y$. On solving, we get $2y=2x$ or $x=y$.
Therefore, $RS=RO+PQ+PS$, we have $RQ=x$, $PQ=10-2x$ and $PS=y$, thus we get $RS=x+10-2x+y$, solving further by taking $x=y$, we get $RS=10$. Thus, the length of RS is 10 and option c) is correct.
Note: The question becomes very easy if we know the property that the length of two tangents from a point to a circle are equal. It can be proved easily using the concept of congruence of triangles.
Complete step by step answer:

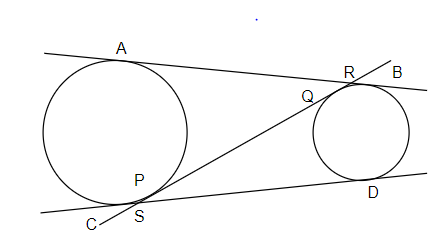
it is given in the question that S1 and S2 are two unequal circles. Also, AB and CD are the direct common tangents to the circles. It is also given that a transverse common tangent PQ cuts AB in R and CD in S, and length of AB=10 then we have to find the length of RS.

We know that the length of two tangents from a point to the circle are of equal length. So, we get $RQ=RB$ and $SC=SP$. Let us assume that $RQ=RB=x$ and $SC=SP=y$. Now, we know that the length of AB is 10 units, so we get length $AR=AB-RB=RP=10-x$. AR and RP are the two tangents from a common point R to the circle S1 thus are of equal length.
As we have $AR=AB-RB=RP=10-x$ and $RQ=x$, so from this we get \[PQ=RP-RQ=10-x-x\] or $PQ=10-2x$. Again the tangents SQ and SD are equal because both are common on point S and circle ${{S}_{2}}$, and also we had assumed that \[SP=y\] and we have already calculated \[PQ=10-2x\]. Therefore, we get \[SQ=SP+PQ=y+10-2x=SD\].
We also know that the length of direct common tangents of two circles are equal thus tangents $AB=CD=10$. Now, we get $CD=CS+SD$ and we have $CD=10,CS=y$ and $SD=10-2x+y$. On putting the value of CD, CS and SD, we get $10=y+10-2x+y$. On solving, we get $2y=2x$ or $x=y$.
Therefore, $RS=RO+PQ+PS$, we have $RQ=x$, $PQ=10-2x$ and $PS=y$, thus we get $RS=x+10-2x+y$, solving further by taking $x=y$, we get $RS=10$. Thus, the length of RS is 10 and option c) is correct.
Note: The question becomes very easy if we know the property that the length of two tangents from a point to a circle are equal. It can be proved easily using the concept of congruence of triangles.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If x be real then the maximum value of 5 + 4x 4x2 will class 10 maths JEE_Main

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE