Answer
462k+ views
Hint: This question is based on geometry only. Here we assume angles $\angle AOC$ and $\angle COB$ according to the ratio of arc $\overset\frown{AC}$ and $\overset\frown{AB}$ given in the question. Then using following properties, we calculate all angles:-
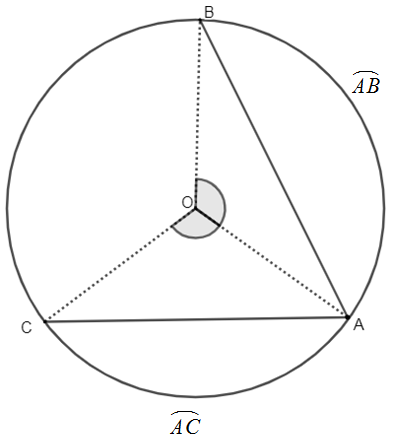
(i) Ratio between two arcs and ratio between angles subtended by corresponding arcs at centre are equal.
$\dfrac{m\overset\frown{AB}}{m\overset\frown{AC}}=\dfrac{m\angle AOB}{m\angle AOC}$
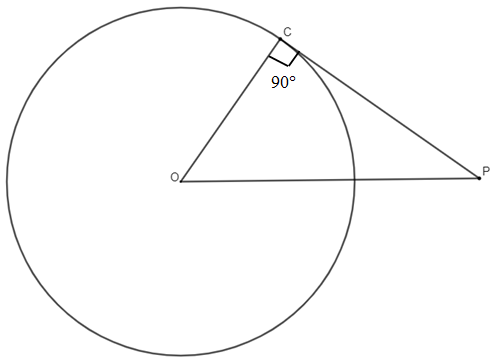
(ii) Angle between tangent and line joining centre and point of contact or tangency is right angle or $90{}^\circ $.
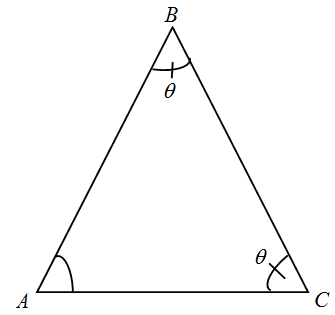
(iii) In any triangle, angles in front of any two equal sides are equal.
$AB=AC$
$\Rightarrow \angle ABC=\angle ACB$
Complete step-by-step answer:
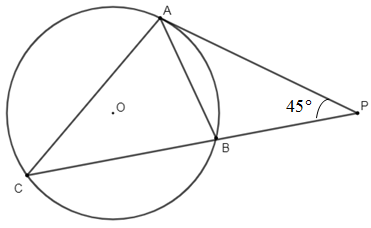
Now, according to the question, $\angle APB=45{}^\circ $ and the ratio between arc $\overset\frown{AC}$ and $\overset\frown{AB}$ is equal to $5:2$.
We know that ratio between length of arcs and ratio between angles subtended by corresponding arcs at centre are equal.
$\dfrac{m\overset\frown{AC}}{m\overset\frown{AB}}=\dfrac{m\angle AOC}{m\angle AOB}$
$\therefore \dfrac{m\overset\frown{AC}}{m\overset\frown{AB}}=\dfrac{m\angle AOC}{m\angle AOB}=\dfrac{5}{2}$
Let us now assume $\angle AOC$ is $5\theta $ and $\angle AOB$ is $2\theta $.
$\therefore ~\angle AOC=5\theta $ and $\angle AOB=2\theta $
Now, we know that, angle between tangent and radius is always $90{}^\circ $. In the figure below, $PA$ is a tangent on the circle and $AO$ is the radius. Hence, $\angle PAO$ is a right angle.
$\therefore \angle PAO=90{}^\circ $
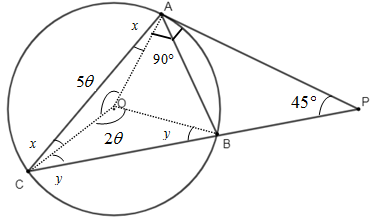
Also, in any triangle angles in front of any two equal sides are equal. So, in$\Delta AOC$, $AO$ and $OC$ are radius. Then,$\angle OAC=\angle OCA$.
And, in$\Delta BOC$, $BO$ and $OC$ are the radius of the circle. So, $BO=OC$. Then, $\angle OBC=\angle OCB$.
Let us assume $\angle OAC=\angle OCA=x{}^\circ $ and $\angle OBC=\angle OCB=y{}^\circ $.
Since the sum of internal angles in any triangle is $180{}^\circ $,in $\Delta AOC$-
$\angle AOC+\angle OAC+\angle OCA=180{}^\circ $
$\Rightarrow 5\theta +x+x=180{}^\circ $
$\Rightarrow 2x=180{}^\circ -5\theta $
\[\Rightarrow x=90{}^\circ -\dfrac{5\theta }{2}\]
And, in $\Delta COB$,
$\angle COB+\angle OBC+\angle OCB=180{}^\circ $
$\Rightarrow 2\theta +y+y=180{}^\circ $
$\Rightarrow 2y=180{}^\circ -2\theta $
$\Rightarrow y=90{}^\circ -\theta $
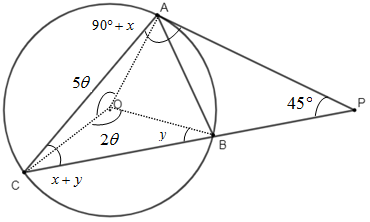
Now, according to figure –
$\angle PAC=90{}^\circ +x$
$\angle ACP=x+y$
$\angle APC=45{}^\circ $
In $\Delta PAC$,
\[\angle PAC+\angle ACP+\angle APC=180{}^\circ \]
$\Rightarrow \left( 90{}^\circ +x \right)+\left( x+y \right)+45{}^\circ =180{}^\circ $
$\Rightarrow 2x+y=45{}^\circ $
$\because x=90{}^\circ -\dfrac{5\theta }{2}$ and $y=90{}^\circ -\theta $
$\Rightarrow 2\left( 90{}^\circ -\dfrac{5\theta }{2} \right)+\left( 90{}^\circ -\theta \right)=45{}^\circ $
$\Rightarrow 180{}^\circ -5\theta +90{}^\circ -\theta =45{}^\circ $
$\Rightarrow 225{}^\circ =6\theta $
$\Rightarrow \theta =\dfrac{225{}^\circ }{6}$
$\Rightarrow \theta =37.5{}^\circ $
So,
$x=90{}^\circ -\dfrac{5\theta }{2}$
$=90{}^\circ -\dfrac{5\times 37.5}{2}$
$=90{}^\circ -\dfrac{187.5{}^\circ }{2}$
$=-3.75{}^\circ $
And,
$y=90{}^\circ -\theta $
$=90{}^\circ -37.5{}^\circ $
$=52.5{}^\circ $
Also, $\angle AOC=5\theta =5\times 37.5=187.5{}^\circ $
$\angle COB=2\theta =2\times 37.5=75{}^\circ $
So, the actual figure will be –
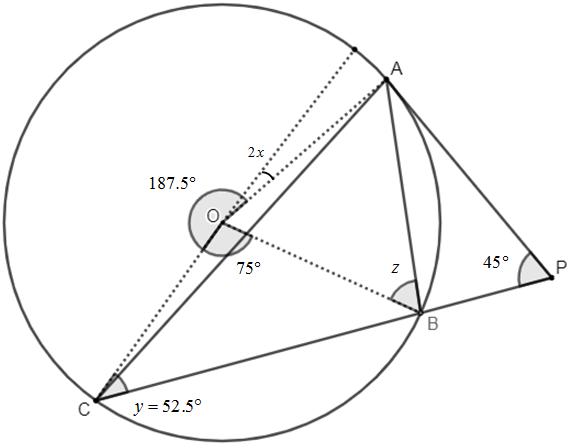
We know that the whole circle subtends an angle of $360{}^\circ $ at the centre.
$\therefore \angle COB+\angle BOA+\angle AOC=360{}^\circ $
$\Rightarrow 75{}^\circ +\angle BOA+187.5{}^\circ =360{}^\circ $
$\Rightarrow \angle BOA=97.5{}^\circ $
Now, $AO$ and $OB$ are the radius of the circle.
$\therefore AO=OB$
We know that in a triangle, angles in front of any two equal sides are equal.
$\Rightarrow \angle OAB=\angle OBA$
Let $\angle OAB=\angle OBA=x{}^\circ $
So, in $\Delta AOB$,
$\angle AOB+\angle OAB+\angle OBA=180{}^\circ $
$\Rightarrow 97.5{}^\circ +z+z=180{}^\circ $
$\Rightarrow 2z=82.5{}^\circ $
$\Rightarrow z=41.25{}^\circ $
$\therefore \angle OAB=\angle OBA=41.25{}^\circ $
Now according to the figure, as $\overline{CBP}$ is a straight line, so
$\angle CBO+\angle OBA+\angle ABP=180{}^\circ $
$\Rightarrow y+z+\angle ABP=180{}^\circ $
$\Rightarrow 52.5{}^\circ +41.25{}^\circ +\angle ABP=180{}^\circ $
$\Rightarrow \angle ABP=86.25{}^\circ $
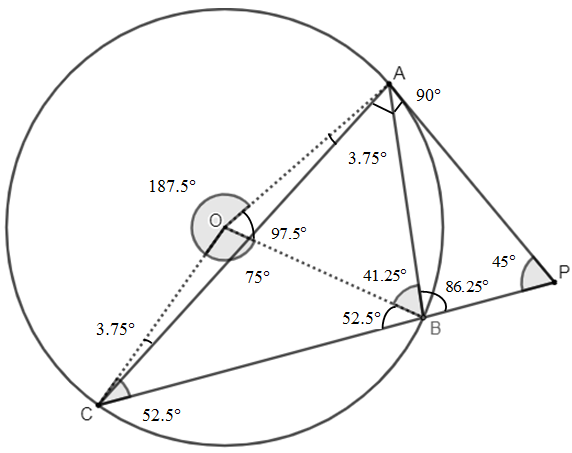
According to the above figure –
$\angle COA=187.5{}^\circ $ (major arc)
$\angle COB=75{}^\circ $ (minor arc)
$\angle BOA=97.5{}^\circ $ (minor arc)
$\angle OCA=3.75{}^\circ =\angle OAC$
$\angle OCB=\angle OBC=52.5{}^\circ $
$\angle OBA=\angle OAB=41.25{}^\circ $
$\angle ABP=86.25{}^\circ $
According to the figure,
$\angle PAC=\angle PAO-\angle CAO$
$\Rightarrow \angle PAC=90{}^\circ -3.75{}^\circ $
$\Rightarrow \angle PAC=86.25{}^\circ $
And,
$\angle ACB=\angle OCB-\angle OCA$
$\Rightarrow \angle ACB=52.5{}^\circ -3.75{}^\circ $
$\Rightarrow \angle ACB=48.75{}^\circ $
Hence, \[m\angle PAC=86.25{}^\circ \]and $\angle ACB=48.75{}^\circ $ .
Note: Students should take care of calculations and all angles carefully. If we take any angle wrong, there will be mistakes in all angle calculations. Here we have to take care of major arcs and minor arcs also.
(i) Ratio between two arcs and ratio between angles subtended by corresponding arcs at centre are equal.
$\dfrac{m\overset\frown{AB}}{m\overset\frown{AC}}=\dfrac{m\angle AOB}{m\angle AOC}$

(ii) Angle between tangent and line joining centre and point of contact or tangency is right angle or $90{}^\circ $.

(iii) In any triangle, angles in front of any two equal sides are equal.
$AB=AC$
$\Rightarrow \angle ABC=\angle ACB$

Complete step-by-step answer:
Now, according to the question, $\angle APB=45{}^\circ $ and the ratio between arc $\overset\frown{AC}$ and $\overset\frown{AB}$ is equal to $5:2$.
We know that ratio between length of arcs and ratio between angles subtended by corresponding arcs at centre are equal.
$\dfrac{m\overset\frown{AC}}{m\overset\frown{AB}}=\dfrac{m\angle AOC}{m\angle AOB}$
$\therefore \dfrac{m\overset\frown{AC}}{m\overset\frown{AB}}=\dfrac{m\angle AOC}{m\angle AOB}=\dfrac{5}{2}$
Let us now assume $\angle AOC$ is $5\theta $ and $\angle AOB$ is $2\theta $.
$\therefore ~\angle AOC=5\theta $ and $\angle AOB=2\theta $
Now, we know that, angle between tangent and radius is always $90{}^\circ $. In the figure below, $PA$ is a tangent on the circle and $AO$ is the radius. Hence, $\angle PAO$ is a right angle.
$\therefore \angle PAO=90{}^\circ $

Also, in any triangle angles in front of any two equal sides are equal. So, in$\Delta AOC$, $AO$ and $OC$ are radius. Then,$\angle OAC=\angle OCA$.
And, in$\Delta BOC$, $BO$ and $OC$ are the radius of the circle. So, $BO=OC$. Then, $\angle OBC=\angle OCB$.
Let us assume $\angle OAC=\angle OCA=x{}^\circ $ and $\angle OBC=\angle OCB=y{}^\circ $.
Since the sum of internal angles in any triangle is $180{}^\circ $,in $\Delta AOC$-
$\angle AOC+\angle OAC+\angle OCA=180{}^\circ $
$\Rightarrow 5\theta +x+x=180{}^\circ $
$\Rightarrow 2x=180{}^\circ -5\theta $
\[\Rightarrow x=90{}^\circ -\dfrac{5\theta }{2}\]
And, in $\Delta COB$,
$\angle COB+\angle OBC+\angle OCB=180{}^\circ $
$\Rightarrow 2\theta +y+y=180{}^\circ $
$\Rightarrow 2y=180{}^\circ -2\theta $
$\Rightarrow y=90{}^\circ -\theta $

Now, according to figure –
$\angle PAC=90{}^\circ +x$
$\angle ACP=x+y$
$\angle APC=45{}^\circ $
In $\Delta PAC$,
\[\angle PAC+\angle ACP+\angle APC=180{}^\circ \]
$\Rightarrow \left( 90{}^\circ +x \right)+\left( x+y \right)+45{}^\circ =180{}^\circ $
$\Rightarrow 2x+y=45{}^\circ $
$\because x=90{}^\circ -\dfrac{5\theta }{2}$ and $y=90{}^\circ -\theta $
$\Rightarrow 2\left( 90{}^\circ -\dfrac{5\theta }{2} \right)+\left( 90{}^\circ -\theta \right)=45{}^\circ $
$\Rightarrow 180{}^\circ -5\theta +90{}^\circ -\theta =45{}^\circ $
$\Rightarrow 225{}^\circ =6\theta $
$\Rightarrow \theta =\dfrac{225{}^\circ }{6}$
$\Rightarrow \theta =37.5{}^\circ $
So,
$x=90{}^\circ -\dfrac{5\theta }{2}$
$=90{}^\circ -\dfrac{5\times 37.5}{2}$
$=90{}^\circ -\dfrac{187.5{}^\circ }{2}$
$=-3.75{}^\circ $
And,
$y=90{}^\circ -\theta $
$=90{}^\circ -37.5{}^\circ $
$=52.5{}^\circ $
Also, $\angle AOC=5\theta =5\times 37.5=187.5{}^\circ $
$\angle COB=2\theta =2\times 37.5=75{}^\circ $
So, the actual figure will be –

We know that the whole circle subtends an angle of $360{}^\circ $ at the centre.
$\therefore \angle COB+\angle BOA+\angle AOC=360{}^\circ $
$\Rightarrow 75{}^\circ +\angle BOA+187.5{}^\circ =360{}^\circ $
$\Rightarrow \angle BOA=97.5{}^\circ $
Now, $AO$ and $OB$ are the radius of the circle.
$\therefore AO=OB$
We know that in a triangle, angles in front of any two equal sides are equal.
$\Rightarrow \angle OAB=\angle OBA$
Let $\angle OAB=\angle OBA=x{}^\circ $
So, in $\Delta AOB$,
$\angle AOB+\angle OAB+\angle OBA=180{}^\circ $
$\Rightarrow 97.5{}^\circ +z+z=180{}^\circ $
$\Rightarrow 2z=82.5{}^\circ $
$\Rightarrow z=41.25{}^\circ $
$\therefore \angle OAB=\angle OBA=41.25{}^\circ $
Now according to the figure, as $\overline{CBP}$ is a straight line, so
$\angle CBO+\angle OBA+\angle ABP=180{}^\circ $
$\Rightarrow y+z+\angle ABP=180{}^\circ $
$\Rightarrow 52.5{}^\circ +41.25{}^\circ +\angle ABP=180{}^\circ $
$\Rightarrow \angle ABP=86.25{}^\circ $

According to the above figure –
$\angle COA=187.5{}^\circ $ (major arc)
$\angle COB=75{}^\circ $ (minor arc)
$\angle BOA=97.5{}^\circ $ (minor arc)
$\angle OCA=3.75{}^\circ =\angle OAC$
$\angle OCB=\angle OBC=52.5{}^\circ $
$\angle OBA=\angle OAB=41.25{}^\circ $
$\angle ABP=86.25{}^\circ $
According to the figure,
$\angle PAC=\angle PAO-\angle CAO$
$\Rightarrow \angle PAC=90{}^\circ -3.75{}^\circ $
$\Rightarrow \angle PAC=86.25{}^\circ $
And,
$\angle ACB=\angle OCB-\angle OCA$
$\Rightarrow \angle ACB=52.5{}^\circ -3.75{}^\circ $
$\Rightarrow \angle ACB=48.75{}^\circ $
Hence, \[m\angle PAC=86.25{}^\circ \]and $\angle ACB=48.75{}^\circ $ .
Note: Students should take care of calculations and all angles carefully. If we take any angle wrong, there will be mistakes in all angle calculations. Here we have to take care of major arcs and minor arcs also.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If x be real then the maximum value of 5 + 4x 4x2 will class 10 maths JEE_Main

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE