Question

The absolute refractive index of water is $\dfrac{4}{3}$. What is the critical angle ?

Answer 1

Hint: Critical angle is defined as the angle of incidence beyond which rays of light passing through a denser medium to the surface of a less dense medium are no longer refracted but totally reflected and numerically it is given as $c = {\sin ^{ - 1}}\left( {\dfrac{{{n_r}}}{{{n_i}}}} \right)$
Where
c $ = $ critical angle
${n_r} = $ refractive index of medium where ray will be refracted
${n_i} = $ refractive index of medium where ray is incident

Complete step by step answer:
The absolute refractive index is defined as the ratio of the speed of light in vacuum and in the given medium. It should never be less than 1.
Given that the absolute refractive index of water is $ = \dfrac{4}{3}$.
Now we have to find critical angle
Critical angle is the angle of incidence beyond which the total internal reflection of light occurs.
Total internal reflection is a phenomenon in which the incident ray becomes completely reflected

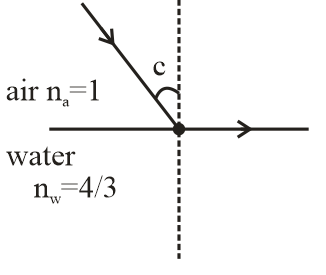
In the diagram, we can easily see that the ray becomes completely reflected. So, the critical angle is given as
$\sin c = \dfrac{{{n_r}}}{{{n_i}}}$
Here ${n_r} = {n_w} = \dfrac{4}{3}$
${n_i} = {n_a} = 1$
So, $\sin c = \dfrac{1}{{4/3}} = \dfrac{3}{4}$
$c = {\sin ^1}\left( {\dfrac{3}{4}} \right)$
$c = 48.5$

Note:
Total internal reflection occurs only when light is travelling from rarer medium to denser medium.
When light travels from rarer to denser medium then it is moving far away from normal.
When light travels from denser to rarer medium then it is moving towards normal.