
The adjacent sides of a parallelogram are
Answer
469.2k+ views
Hint: Here, we will first find the area of the parallelogram with the shorter side as the base and the given perpendicular distance as the height. Then we will find the area using the longer side as the base and the distance between longer sides as the height. We will then equate these areas and solve further to find the required distance between the longer sides.
Formula Used:
Area of parallelogram
Complete step-by-step answer:
In a parallelogram, opposite sides are parallel and equal.
It is given that the adjacent sides of a parallelogram are
The longer side,
The shorter side,
Now, the perpendicular distance between the shorter sides is
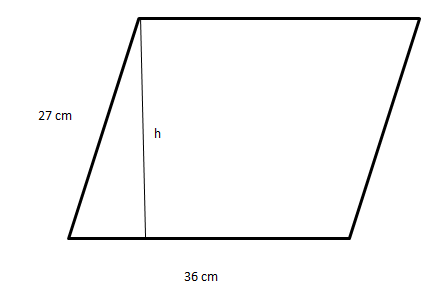
We will now find the area of the parallelogram.
In this case, the base is the shorter side, i.e.
Therefore using the formula of area of parallelogram
Now, if we consider the base as the longer side, i.e.
Therefore using the formula of area of parallelogram
We know that both the areas are equal because they are the areas of the same parallelogram.
Hence, equating both the areas, we get
Dividing both side by 36, we get
Simplifying the expression, we get
Therefore, the distance between the longer sides,
Thus, this is the required answer.
Note: A parallelogram is a quadrilateral in which the pair of opposite sides are parallel and equal to each other. Also, each diagonal in a parallelogram divides it into two congruent triangles. The diagonals of the parallelogram bisect each other and divide each other into two equal parts. This means that if the length of the whole diagonal is for example 6 cm then, after intersecting with another diagonal, it gets divided into two equal parts of 3 cm each.
Formula Used:
Area of parallelogram
Complete step-by-step answer:
In a parallelogram, opposite sides are parallel and equal.
It is given that the adjacent sides of a parallelogram are
The longer side,
The shorter side,
Now, the perpendicular distance between the shorter sides is

We will now find the area of the parallelogram.
In this case, the base is the shorter side, i.e.
Therefore using the formula of area of parallelogram
Now, if we consider the base as the longer side, i.e.
Therefore using the formula of area of parallelogram
We know that both the areas are equal because they are the areas of the same parallelogram.
Hence, equating both the areas, we get
Dividing both side by 36, we get
Simplifying the expression, we get
Therefore, the distance between the longer sides,
Thus, this is the required answer.
Note: A parallelogram is a quadrilateral in which the pair of opposite sides are parallel and equal to each other. Also, each diagonal in a parallelogram divides it into two congruent triangles. The diagonals of the parallelogram bisect each other and divide each other into two equal parts. This means that if the length of the whole diagonal is for example 6 cm then, after intersecting with another diagonal, it gets divided into two equal parts of 3 cm each.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Write a pair of integers whose sum gives i Zero ii class 7 maths CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE





