
The adjacent sides of a rectangle are
Answer
523.2k+ views
Hint: If we have the length and breadth of a rectangle, then its area is equal to the product of its length and breadth. Mathematically, it can be written as follows:
Area of rectangle = length (l)
Complete step-by-step answer:
In the question, we have been given that the adjacent sides of a rectangle are
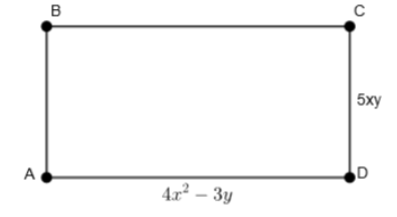
The longest side in the figure represents the length of the rectangle and it is taken in the horizontal direction. So, we get the length of the rectangle as
So, let us represent the dimensions as length,
Now, we know that area
So we can substitute the values of l and b in it and we get,
On further multiplying the terms, we get,
Therefore, the area of the rectangle is equal to
Note: Students have to be careful while calculating the area of the rectangle. Also, students need to remember that the adjacent sides of a rectangle are length and breadth. Also, don’t forget to mention the unit of the area in the answer. Since the area is in the space occupied by a flat surface or the surface of an object, it is measured in the number of unit squares that cover the surface.
Area of rectangle = length (l)
Complete step-by-step answer:
In the question, we have been given that the adjacent sides of a rectangle are

The longest side in the figure represents the length of the rectangle and it is taken in the horizontal direction. So, we get the length of the rectangle as
So, let us represent the dimensions as length,
Now, we know that area
So we can substitute the values of l and b in it and we get,
On further multiplying the terms, we get,
Therefore, the area of the rectangle is equal to
Note: Students have to be careful while calculating the area of the rectangle. Also, students need to remember that the adjacent sides of a rectangle are length and breadth. Also, don’t forget to mention the unit of the area in the answer. Since the area is in the space occupied by a flat surface or the surface of an object, it is measured in the number of unit squares that cover the surface.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




