Question

The altitude of the frustum of a regular rectangular pyramid is 5 m, the volume is 140 cu. m. and the upper base is 3 m and 4 m. What are the dimensions of the lower base in m?
( a ) 9 $\times $ 10
( b ) 6 $\times $ 8
( c ) 4.5 $\times $ 6
( d ) 7.5 $\times $ 10

Answer 1

Hint: Frustum is a portion of the pyramid which remains after the upper part is cut off, that is we have a shape such that planes on top of cones as well as the bottom of the pyramid.
And the, volume of Frustum = \[\dfrac{h}{3}\left( {{U}_{1}}+{{U}_{2}}+\sqrt{({{U}_{1}}\times {{U}_{2}})} \right)\], where, h = is height of frustum, ${{U}_{1}}$ is upper base of frustum, ${{U}_{2}}$ is lower base of frustum. So, in this question we will find the area of the upper plane of frustum then we will put all values in the formula of volume and evaluate the area of the lower plane of frustum.

Complete step-by-step answer:

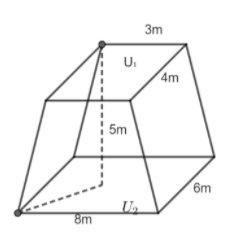
Let, height of pyramid be h, volume be V, upper base be ${{U}_{1}}$ and lower base be ${{U}_{2}}$ , length of upper base be L, breadth be B, length of lower base be l, breadth be b.
We are given that height of pyramid ( h ) =5 m, Volume ( V ) = 140 cu. m.,
Length of upper base ( L ) = 3m, breadth ( B ) = 4 m
So, the area of upper base ${{U}_{1}}$ = L $\times $ B = 3 $\times $ 4 = 12 ${{m}^{2}}$ .
and, area of lower base ${{U}_{2}}$ = ( l $\times $ b ) ${{m}^{2}}$
We know that the, Volume of frustum ( V ) = \[\dfrac{\pi h}{3}\left( {{U}_{1}}+{{U}_{2}}+\sqrt{({{U}_{1}}\times {{U}_{2}})} \right)\]……( i )
where, h = is height of frustum, ${{U}_{1}}$ is upper base of frustum, ${{U}_{2}}$ is lower base of frustum.
Putting values of volume V, height h, upper base ${{U}_{1}}$, lower base ${{U}_{2}}$in ( i )
\[\begin{align}
  & 140=\dfrac{5}{3}(12+{{U}_{2}}+\sqrt{(12\times {{U}_{2}})}) \\
 & 84=({{U}_{2}}+12+\sqrt{(12\times {{U}_{2}})}) \\
 & {{U}_{2}}+\sqrt{(12\times {{U}_{2}})}-72=0 \\
\end{align}\]
Adding and subtracting $\dfrac{12}{4}$ in equation ( i ), we get
\[{{U}_{1}}+\dfrac{12}{4}-\dfrac{12}{4}+\sqrt{(12\times {{U}_{2}})}-72=0\]
\[\left( {{U}_{1}}+\dfrac{12}{4}+\sqrt{(12\times {{U}_{2}})} \right)-\dfrac{12}{4}-72=0\]
\[\begin{align}
  & {{\left( \sqrt{{{U}_{2}}}+\dfrac{\sqrt{12}}{2} \right)}^{2}}-\dfrac{12}{4}-72=0 \\
 & \\
\end{align}\]
Solving further we get,
\[\begin{align}
  & {{\left( \sqrt{{{U}_{2}}}+\dfrac{\sqrt{12}}{2} \right)}^{2}}=75 \\
 & \left( \sqrt{{{U}_{2}}}+\dfrac{\sqrt{12}}{2} \right)=\sqrt{75} \\
 & \left( \sqrt{{{U}_{2}}}+2\sqrt{3} \right)=5\sqrt{3} \\
 & \left( \sqrt{{{U}_{2}}} \right)=4\sqrt{3} \\
 & {{U}_{2}}={{(4\sqrt{3})}^{2}} \\
 & {{U}_{2}}=48{{m}^{2}} \\
\end{align}\]
Now, 48 \[{{m}^{2}}\] = 6 m $\times $ 8 m
Hence, option ( b ) is correct.

Note: Other than completing the square method you can use another method to evaluate the value of lower plane of frustum. Calculation can be complex and lengthy so simplification must be accurate else the answer can get incorrect. Units should be kept mentioned in every solution.