
The angle between two vectors
A.)
B.)
C.)
D.
Answer
514.5k+ views
1 likes
Hint – You can start the solution by drawing a well-labelled diagram with all the vectors (
Complete answer:
To solve this equation, consider the diagram given below
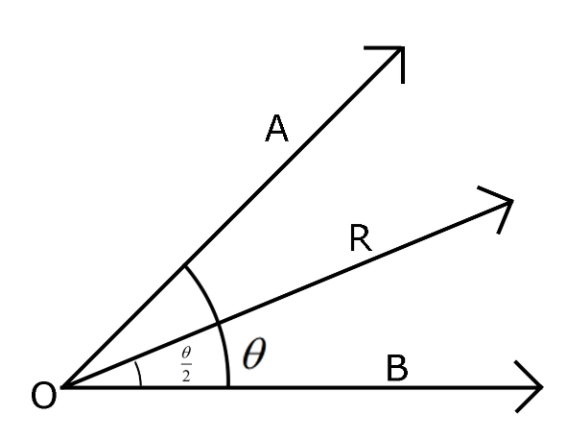
The arrangement of
We know,
And
We also know,
The equation for the
Hence, Option C is the correct option
Additional Information:
A vector is a mathematical quantity that has both a magnitude (size) and a direction. To imagine what a vector is like, imagine asking someone for directions in an unknown area and they tell you, “Go
A vector quantity is different from a scalar quantity in the fact that a scalar quantity has only magnitude, but a vector quantity possesses both direction and magnitude. Unlike scalar quantities, vector quantities cannot undergo any mathematical operation, instead they undergo Dot product and Cross product.
Some examples of vectors are – Displacement, Force, Acceleration, Velocity, Momentum, etc.
Note – You can also get to the solution by not going through the mathematical calculations and just focusing on the theoretical part. You can make an argument that we know that
Complete answer:
To solve this equation, consider the diagram given below

The arrangement of
We know,
And
We also know,
The equation for the
Hence, Option C is the correct option
Additional Information:
A vector is a mathematical quantity that has both a magnitude (size) and a direction. To imagine what a vector is like, imagine asking someone for directions in an unknown area and they tell you, “Go
A vector quantity is different from a scalar quantity in the fact that a scalar quantity has only magnitude, but a vector quantity possesses both direction and magnitude. Unlike scalar quantities, vector quantities cannot undergo any mathematical operation, instead they undergo Dot product and Cross product.
Some examples of vectors are – Displacement, Force, Acceleration, Velocity, Momentum, etc.
Note – You can also get to the solution by not going through the mathematical calculations and just focusing on the theoretical part. You can make an argument that we know that
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




