
The angle of elevation of the top of a tower at a distance of
Answer
505.2k+ views
Hint: This is a question of Trigonometry from the Height and Distance section. To solve this we will use a formula of trigonometric identity applicable in a right angle triangle. Here first we should observe which trigonometric identity we can use here , suppose if we have perpendicular and base then we will use
Suppose in a right angle triangle if one angle is
Then we know formula of
Complete step-by-step answer:
Let’s assume height of the tower
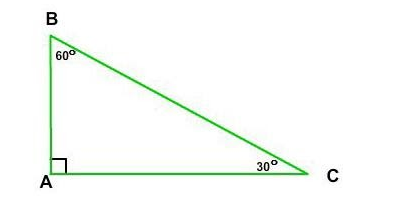
Here, AB represents the height of the tower and AC is horizontal distance.
We have to find value of
Put the value of
Height of tower is
Note: To solve this question we should have knowledge of trigonometric identity and their formulas, we should know which trigonometric identity is applicable here among all six. We should know the concept of elevation and depression angles too. If we are looking at something from downward to upward then our eyes make an elevation angle but if we are looking from upward to downward then our eyes make a depressed angle.
Suppose in a right angle triangle if one angle is
Then we know formula of
Complete step-by-step answer:
Let’s assume height of the tower

Here, AB represents the height of the tower and AC is horizontal distance.
We have to find value of
Put the value of
Height of tower is
Note: To solve this question we should have knowledge of trigonometric identity and their formulas, we should know which trigonometric identity is applicable here among all six. We should know the concept of elevation and depression angles too. If we are looking at something from downward to upward then our eyes make an elevation angle but if we are looking from upward to downward then our eyes make a depressed angle.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




