
The angle of elevation of the top of the tower as observed from a point in the horizontal plane through the foot of the tower is
Answer
524.7k+ views
Hint: Assume that the height of the tower is h. Using
Using
Complete step-by-step answer:
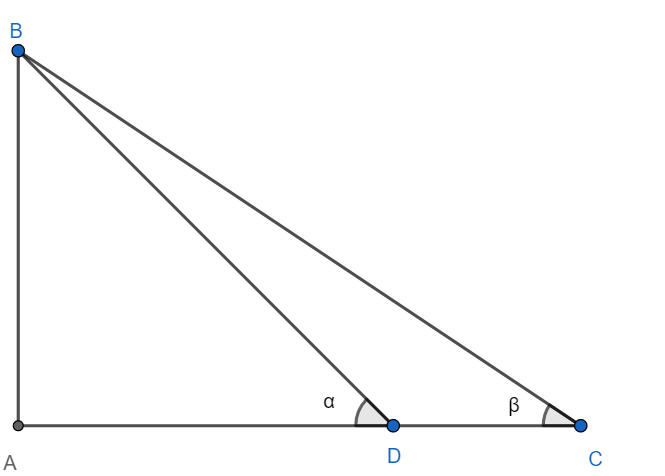
Given: AB is a tower. The angle of elevation of the tower from point C is
To determine: The height of the tower AB and the distance of C from the foot of the tower.
Let the height of the tower be h. Hence, we have AB = h.
We have in triangle ABD, AB is the side opposite to
We know that
Hence, we have
Multiplying both sides by
Also, in triangle ABC, AB is the side opposite to
We know that
Hence, we have
Multiplying both sides by AC, we get
Also, we have AC = AD +DC
Substituting the value of AD from equation (i) and substituting DC = 100, we get
Hence, we have
Subtracting
Taking
Dividing both sides of the equation by
Substituting
Hence, we have h = 91.67m
Hence the height of the tower is 91.67m.
Substituting the value of h in the equation (i), we get
Hence, we have AC = AD + DC = 46.7+100 = 146.7m.
Hence the distance of the first point of observation from the foot of the tower is 146.7 m.
Note: Verification:
We have AB = 91.67m, AD = 46.7m
Hence, we have
Hence
Also, AB = 91.67m and AC = 146.7m
Hence, we have
Hence, we have
Hence our solution is verified to be correct.
Using
Complete step-by-step answer:

Given: AB is a tower. The angle of elevation of the tower from point C is
To determine: The height of the tower AB and the distance of C from the foot of the tower.
Let the height of the tower be h. Hence, we have AB = h.
We have in triangle ABD, AB is the side opposite to
We know that
Hence, we have
Multiplying both sides by
Also, in triangle ABC, AB is the side opposite to
We know that
Hence, we have
Multiplying both sides by AC, we get
Also, we have AC = AD +DC
Substituting the value of AD from equation (i) and substituting DC = 100, we get
Hence, we have
Subtracting
Taking
Dividing both sides of the equation by
Substituting
Hence, we have h = 91.67m
Hence the height of the tower is 91.67m.
Substituting the value of h in the equation (i), we get
Hence, we have AC = AD + DC = 46.7+100 = 146.7m.
Hence the distance of the first point of observation from the foot of the tower is 146.7 m.
Note: Verification:
We have AB = 91.67m, AD = 46.7m
Hence, we have
Hence
Also, AB = 91.67m and AC = 146.7m
Hence, we have
Hence, we have
Hence our solution is verified to be correct.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




