
The angle of incidence in air for a light ray is 40 degrees. If the ray travels through water of refractive index 4/3. Find the angle of refraction.
(
Answer
488.7k+ views
Hint: The relationship of angle of incidence and angle of refraction at interface of two media is given by Snell’s law. Snell’s law states that the ratio of the sines of the angles of incidence and refraction is equal to the reciprocal of the ratio of the indices of refraction.
Use Snell’s law to find the angle of refraction.
Formula used:
Law of refraction or Snell’s law,
Complete step-by-step answer:
When a light wave passes at some angle from one medium to another medium, its direction changes. This phenomenon is referred to as refraction.
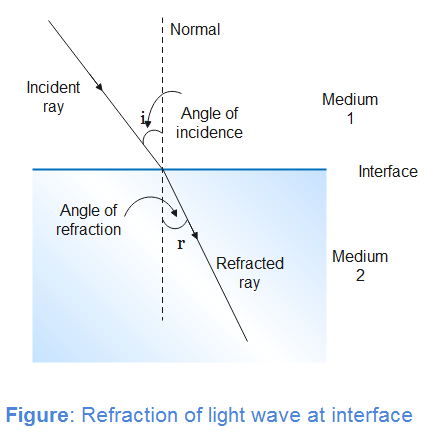
Angle of incidence is defined as the angle between incident ray and normal to the interface of the media and angle of refraction is the angle between refracted ray and normal as shown in figure.
Angle of incidence and angle of refraction are related by Snell’s law. Snell’s law states that the ratio of the sines of the angles of incidence and refraction is equal to the reciprocal of the ratio of the indices of refraction.
We are given that angle of incidence
On rearranging and solving the above equation, we get
Therefore, angle of refraction is
Note: The law of refraction or Snell’s law is valid for all pairs of media at the point of incidence. Incident ray, normal and refracted ray lie in the same plane.
Refractive index of a medium is a unitless quantity. It is also defined as the ratio of velocity of wave in the medium to velocity of wave in vacuum. Refractive index of air or free space is unity.
Use Snell’s law to find the angle of refraction.
Formula used:
Law of refraction or Snell’s law,
Complete step-by-step answer:
When a light wave passes at some angle from one medium to another medium, its direction changes. This phenomenon is referred to as refraction.

Angle of incidence is defined as the angle between incident ray and normal to the interface of the media and angle of refraction is the angle between refracted ray and normal as shown in figure.
Angle of incidence and angle of refraction are related by Snell’s law. Snell’s law states that the ratio of the sines of the angles of incidence and refraction is equal to the reciprocal of the ratio of the indices of refraction.
We are given that angle of incidence
On rearranging and solving the above equation, we get
Therefore, angle of refraction is
Note: The law of refraction or Snell’s law is valid for all pairs of media at the point of incidence. Incident ray, normal and refracted ray lie in the same plane.
Refractive index of a medium is a unitless quantity. It is also defined as the ratio of velocity of wave in the medium to velocity of wave in vacuum. Refractive index of air or free space is unity.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




