
The angle of rotational symmetry for a shape is
[a] 6
[b] 4
[c] 3
[d] 8
Answer
514.5k+ views
Hint:Use the fact that if the angle of rotational symmetry is
Complete step-by-step answer:
Consider an object with the angle of rotational symmetry as x.
Hence, if we rotate the object by x degrees, the shape will remain unchanged.
Now, if we rotate the rotated image again by x degrees, again the net-shape will remain unchanged.
Hence if we rotate the original shape by 2x degrees, the shape will remain unchanged.
Continuing this way if we rotate the image by nx degrees, where n is a natural number, then the shape will remain unchanged.
Now, we know that the order of rotational symmetry of the shape is the number of times it can be rotated around a circle and still look the same.
Let the above shape be rotated n times and still look the same in a complete rotation.
Hence, we have
Dividing both sides by x, we get
Hence, we have
Hence, the order of the rotational symmetry, when the rotational angle of symmetry is
Hence option [a] is correct.
Note: Verification:
A figure with a rotational angle of symmetry as 60 degrees is a regular hexagon.
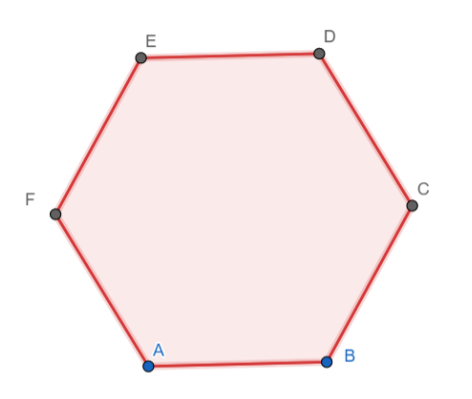
Rotating clockwise by 60 degrees gives the following hexagon
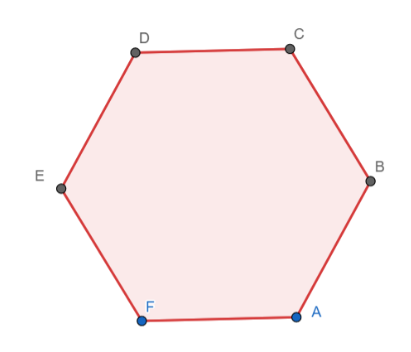
Clearly, the shape remains the same.
We can do the above process 4 more times till a complete rotation is achieved.
Hence the order of rotational symmetry is 6
Complete step-by-step answer:
Consider an object with the angle of rotational symmetry as x.
Hence, if we rotate the object by x degrees, the shape will remain unchanged.
Now, if we rotate the rotated image again by x degrees, again the net-shape will remain unchanged.
Hence if we rotate the original shape by 2x degrees, the shape will remain unchanged.
Continuing this way if we rotate the image by nx degrees, where n is a natural number, then the shape will remain unchanged.
Now, we know that the order of rotational symmetry of the shape is the number of times it can be rotated around a circle and still look the same.
Let the above shape be rotated n times and still look the same in a complete rotation.
Hence, we have
Dividing both sides by x, we get
Hence, we have
Hence, the order of the rotational symmetry, when the rotational angle of symmetry is
Hence option [a] is correct.
Note: Verification:
A figure with a rotational angle of symmetry as 60 degrees is a regular hexagon.

Rotating clockwise by 60 degrees gives the following hexagon

Clearly, the shape remains the same.
We can do the above process 4 more times till a complete rotation is achieved.
Hence the order of rotational symmetry is 6
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




