
The angles of depression of top and bottom of a building
top of a tower are
the horizontal distance between the building and the tower.
Answer
530.7k+ views
Hint: The given question is related to heights and distance. Try to recall Try to recall the formulae
related to trigonometric ratios and values of trigonometric functions for standard angles.
The following formulae will be used to solve the given problem:
(a)
(b)
(c)
Complete step by step solution:
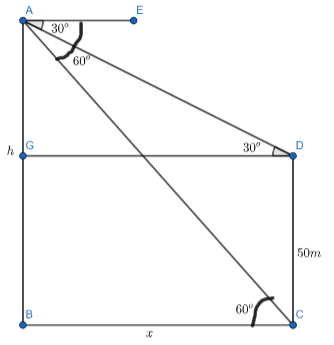
Now, considering the information given in the question, we can draw the following figure for better visualization of the problem:
Let
meter be the horizontal distance between the building and the tower. We will consider a point
on the tower
depression of top and bottom of the building as seen from the top of the tower are
Now,
also be equal. So,
Now, we will consider
In
Now, we will consider
In
Now, the distance between the tower and the building is the same in both cases. So,
On cross-multiplying, we get:
Substituting
Hence, the height of the tower is
to
Note: Students are generally confused between the values of
related to trigonometric ratios and values of trigonometric functions for standard angles.
The following formulae will be used to solve the given problem:
(a)
(b)
(c)
Complete step by step solution:

Now, considering the information given in the question, we can draw the following figure for better visualization of the problem:
Let
meter be the horizontal distance between the building and the tower. We will consider a point
on the tower
depression of top and bottom of the building as seen from the top of the tower are
Now,
also be equal. So,
Now, we will consider
In
Now, we will consider
In
Now, the distance between the tower and the building is the same in both cases. So,
On cross-multiplying, we get:
Substituting
Hence, the height of the tower is
to
Note: Students are generally confused between the values of
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




