
The area of a square is 49 sq cm. A rectangle has the same perimeter as the square. If the length of the rectangle is 9.3 cm, What is its breadth? Also, find which has a greater area?
Answer
500.1k+ views
Hint: Here we will first let the breadth of the rectangle to be b. Now we will apply the formula of the area of the square to find its side and then apply the formula of the perimeter of the square and put it equal to the perimeter of the rectangle to find the breadth.
Now in order to find which has a greater area, we will find the area of rectangle using its formula and then compare the areas of both the figures.
Complete Step-by-step Solution
Let the breadth of the rectangle be
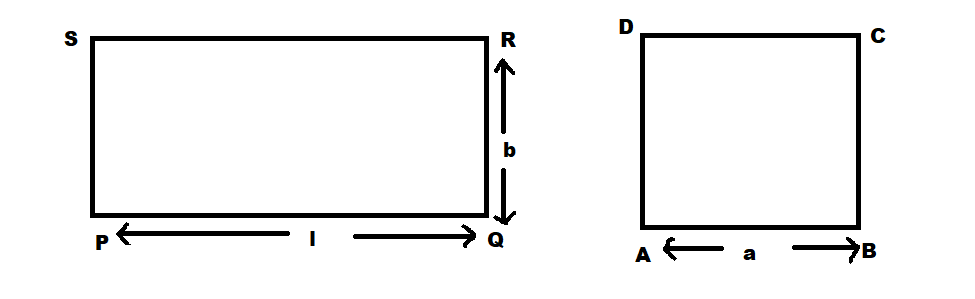
Now since it is given that the area of the square is 49 sq cm.
And we know that the area of the square is given by:
area
Hence putting in the value we get:-
Hence the length of the side of the square is 7cm
Now we will find the perimeter of the square.
Since we know that the perimeter of the square is given by:-
perimeter
Therefore putting in the value of
perimeter
perimeter
Now since it is given that the perimeters of the square and the rectangle are equal
And we know that the perimeter of the rectangle is given by:
perimeter
Hence putting in the known values we get:-
On simplification,
On further simplification,
Hence the breadth of the rectangle is
Now we will calculate the area of the rectangle
Now as we know that the area of the rectangle is given by:-
area
Putting in the known values we get:-
area
area
Now the area of the square is 49 sq cm.
And the area of the rectangle is 43.71 sq cm.
Note:
Students many times make mistakes while multiplying to matrices, they should always keep in mind that while multiplying two matrices we multiply respective elements moving from left to right in rows and from top to bottom in columns. Also, the diagonal entries should be written in a way that they go from top left to right bottom and not from top right to left bottom.
Now in order to find which has a greater area, we will find the area of rectangle using its formula and then compare the areas of both the figures.
Complete Step-by-step Solution
Let the breadth of the rectangle be

Now since it is given that the area of the square is 49 sq cm.
And we know that the area of the square is given by:
area
Hence putting in the value we get:-
Hence the length of the side of the square is 7cm
Now we will find the perimeter of the square.
Since we know that the perimeter of the square is given by:-
perimeter
Therefore putting in the value of
perimeter
perimeter
Now since it is given that the perimeters of the square and the rectangle are equal
And we know that the perimeter of the rectangle is given by:
perimeter
Hence putting in the known values we get:-
On simplification,
On further simplification,
Hence the breadth of the rectangle is
Now we will calculate the area of the rectangle
Now as we know that the area of the rectangle is given by:-
area
Putting in the known values we get:-
area
area
Now the area of the square is 49 sq cm.
And the area of the rectangle is 43.71 sq cm.
Note:
Students many times make mistakes while multiplying to matrices, they should always keep in mind that while multiplying two matrices we multiply respective elements moving from left to right in rows and from top to bottom in columns. Also, the diagonal entries should be written in a way that they go from top left to right bottom and not from top right to left bottom.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Give me the opposite gender of Duck class 8 english CBSE

Advantages and disadvantages of science




