
The area of region bounded by the parabola
A.
B.
C.
D.
Answer
440.1k+ views
Hint: Plot the parabola & draw the line of latus. Find the point of intersection and integrate the curve i.e.
Complete step by step solution:
Compare the given parabola
with
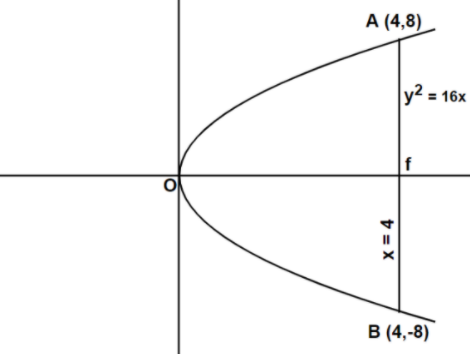
we get
Equation of latus rectum
For
That is,
For
Now Area of OAFB
Additional information:
In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped.
A parabola can also be defined geometrically as a set of points (locus of points) in the
A parabola is a set of points, such that for any point P of the set the distance |PF| to a fixed point F, the focus, is equal to the distance |Pl| to a fixed line l, the directrix
For a parabola, the semi-latus rectum, p, is the distance of the focus from the directrix. Using the parameter p, the equation of the parabola can be rewritten as
In the case of f<0 the parabola has a downward opening.
The presumption that the axis is parallel to the y axis allows one to consider a parabola as the graph of a polynomial of degree 2, and conversely: the graph of an arbitrary polynomial of degree 2 is a parabola (see next section).
If one exchanges x and y, one obtains equations of the form
We can find the area of the region along the y-axis rather than x-axis. In that case function should be in terms of y & limits also for variable y.
Note: In symmetrical diagram or function if it is even function then the answer will be zero so to avoid that we must double the area. The knowledge about the parabola that is the equation of parabola which is
Complete step by step solution:
Compare the given parabola
with

we get
Equation of latus rectum
For
That is,
For
Now Area of OAFB
Additional information:

In mathematics, a parabola is a plane curve which is mirror-symmetrical and is approximately U-shaped.
A parabola can also be defined geometrically as a set of points (locus of points) in the
A parabola is a set of points, such that for any point P of the set the distance |PF| to a fixed point F, the focus, is equal to the distance |Pl| to a fixed line l, the directrix
For a parabola, the semi-latus rectum, p, is the distance of the focus from the directrix. Using the parameter p, the equation of the parabola can be rewritten as
In the case of f<0 the parabola has a downward opening.
The presumption that the axis is parallel to the y axis allows one to consider a parabola as the graph of a polynomial of degree 2, and conversely: the graph of an arbitrary polynomial of degree 2 is a parabola (see next section).
If one exchanges x and y, one obtains equations of the form
We can find the area of the region along the y-axis rather than x-axis. In that case function should be in terms of y & limits also for variable y.
Note: In symmetrical diagram or function if it is even function then the answer will be zero so to avoid that we must double the area. The knowledge about the parabola that is the equation of parabola which is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




