
The area of the quadrilaterals, the coordinates of whose vertices are (1,2), (6,2), (5,3) and (3,4) are
A.
B.5
C.
D.11
Answer
486.6k+ views
Hint: In this question, first we need to draw a quadrilateral ABCD divided in two triangles by making diagonal. After that we will apply the area of triangle formula in both the triangles and in the end, we will find the sum of both the areas. Let the vertices of the quadrilateral be A(1,2),B (6,2), C(5,3) and D(3,4) join BD to form two triangles
As we know that,
Area of triangle with coordinates
Complete step-by-step answer:
Let us divide the quadrilateral ABCD into two triangles ABD and BCD.
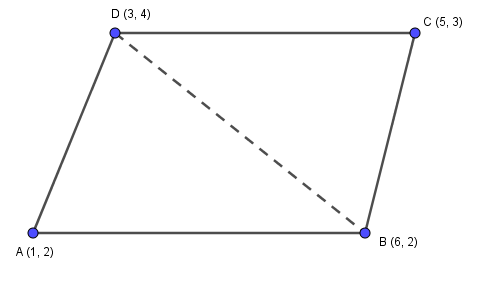
Now, by using the formula.
Area of triangle with coordinates or vertices
Area of triangle ABD with coordinates A (1,2), B (6,2) and D (3,4).
Again, Area of triangle BCD with coordinates B (6,2), C (5,3) and D (3,4).
Now, Area of quadrilateral ABCD = Area of triangle ABD + Area of triangle BCD
= 5 sq. units + 0.5 sq. units
= 5.5 sq. units.
Note: In this question we need to calculate the area of two triangles. So that’s why we used triangle formulas. Also, we have used the concept of quadrilateral so we can also define it: A quadrilateral is a plane figure that has four sides or edges, and also has four corners or vertices. Quadrilateral with four sides like rectangle, rhombus, square, trapezoid, parallelogram, trapezium and kite. In a quadrilateral total interior angle is 360
As we know that,
Area of triangle with coordinates
Complete step-by-step answer:
Let us divide the quadrilateral ABCD into two triangles ABD and BCD.

Now, by using the formula.
Area of triangle with coordinates or vertices
Area of triangle ABD with coordinates A (1,2), B (6,2) and D (3,4).
Again, Area of triangle BCD with coordinates B (6,2), C (5,3) and D (3,4).
Now, Area of quadrilateral ABCD = Area of triangle ABD + Area of triangle BCD
= 5 sq. units + 0.5 sq. units
= 5.5 sq. units.
Note: In this question we need to calculate the area of two triangles. So that’s why we used triangle formulas. Also, we have used the concept of quadrilateral so we can also define it: A quadrilateral is a plane figure that has four sides or edges, and also has four corners or vertices. Quadrilateral with four sides like rectangle, rhombus, square, trapezoid, parallelogram, trapezium and kite. In a quadrilateral total interior angle is 360
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




