
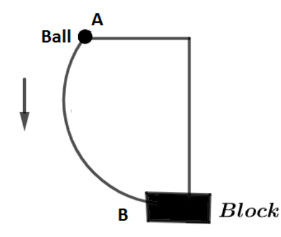
The ball is released when the string is horizontal. The collision between the ball and block is head on elastic. Find out velocities of the ball and the block immediately after the collision.
A.
B.
C.
D. None of these

Answer
424.2k+ views
Hint:In order to solve this equation, we will use the principle of conservation of energy which states that “the total energy of a body is remains constant at any point of the motion of a body such that
Formula used:
Linear momentum of a body is given by
Complete step by step answer:
Now, when the ball is just released it has a potential energy of
So,
Now, just before the head on collision, the initial velocity of ball is
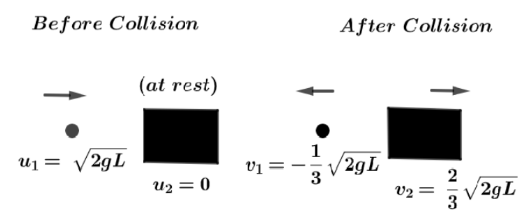
Initial momentum of system
Now, it’s given that the collision is head on elastic. A head on elastic collision is one in which the velocity of approach is equal to the velocity of separation between two bodies.
Velocity of approach is
Velocity of separation is
Velocity of approach is
Now, Adding equation
Now, put this value of
So, the final velocity of ball is
Hence, the correct option is A.
Note: It should be remembered that, when ball just came to collide the block its whole potential energy will convert into kinetic energy and the negative sign of the final velocity of the ball which is
Formula used:
Linear momentum of a body is given by
Complete step by step answer:
Now, when the ball is just released it has a potential energy of
So,
Now, just before the head on collision, the initial velocity of ball is

Initial momentum of system
Now, it’s given that the collision is head on elastic. A head on elastic collision is one in which the velocity of approach is equal to the velocity of separation between two bodies.
Velocity of approach is
Velocity of separation is
Velocity of approach is
Now, Adding equation
Now, put this value of
So, the final velocity of ball is
Hence, the correct option is A.
Note: It should be remembered that, when ball just came to collide the block its whole potential energy will convert into kinetic energy and the negative sign of the final velocity of the ball which is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




