Question

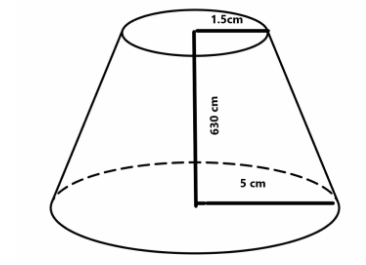
The base and top radius of a truncated cone is 5cm and 1.5cm. And its height is 630 cm. What is the volume of a truncated cone?

Answer 1

Hint: The volume of a cone is given by
   $
  V = \dfrac{1}{3}(\pi {r^2}h) \;
  $ .
In our case it is given that the cone is truncated and the values of top and bottom radius are given.
To calculate the volume of a truncated cone we can use the formula.
 \[V{\text{ }} = \;\dfrac{{\pi h}}{3}({R^2} + Rr + {r^2})\]

Complete step-by-step answer:
Given in the question,
Base radius (R)= 5cm, upper radius(r) = 1.5cm, height(h) = 630 cm
To find the volume we have the formula
\[V{\text{ }} = \;\dfrac{{\pi h}}{3}({R^2} + Rr + {r^2})\]
Substituting the values
 \[
  V{\text{ }} = \;\dfrac{{\pi (630)}}{3} \times [{(5)^2} + (5)(1.5) + {(1.5)^2}] \\
   \Rightarrow 210\pi \times (25 + 7.5 + 2.25) \\
   \Rightarrow 210\pi \times (34.75) \;
 \]
By further solving we get Volume of the truncated cone = $ 22935\,c{m^3} $
So, the correct answer is “ $ 22935\,c{m^3} $ ”.

Note: The truncated cone is the cone without a tip and actually has some radius at top. The formulas to calculate the properties are different from the cone itself. Students must know the formulas for some standard 3D solid figures for a faster and smoother approach.