
The boundary of the shaded region in the adjoining figure is

A. 200 mm
B. 196 mm
C. 186 mm
D. 176 mm

Answer
485.4k+ views
Hint:
The given figure consists of three semicircles. We will first find the radius of the semicircles and then we will compute the circumference of the semicircle using the formula, as
Complete step by step solution:
Here, in the figure, there are three semicircles, where diameter of one semicircle is 56 cm and the diameter of the other two circles is 28 cm.
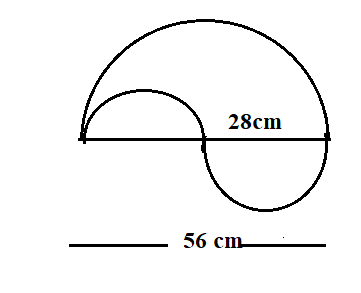
We will first calculate the circumference of all three circles.
Radius is half the diameter of the circle.
Hence, the radius of one semicircle with diameter as 56 cm as
Also, the formula for the circumference of the semicircle is given as
Similarly, the radius of semicircle with diameter as 28 cm as
the boundary of third semicircle will also be 44mm
Hence, the boundary of the shaded region is
Thus, option D is correct.
Note:
Circumference or perimeter gives the length of the boundary of that shape. Hence, we have calculated circumference of the semicircle. Also, when we find the circumference of the semicircle, it does not include the length of its diameter.
The given figure consists of three semicircles. We will first find the radius of the semicircles and then we will compute the circumference of the semicircle using the formula, as
Complete step by step solution:
Here, in the figure, there are three semicircles, where diameter of one semicircle is 56 cm and the diameter of the other two circles is 28 cm.

We will first calculate the circumference of all three circles.
Radius is half the diameter of the circle.
Hence, the radius of one semicircle with diameter as 56 cm as
Also, the formula for the circumference of the semicircle is given as
Similarly, the radius of semicircle with diameter as 28 cm as
the boundary of third semicircle will also be 44mm
Hence, the boundary of the shaded region is
Thus, option D is correct.
Note:
Circumference or perimeter gives the length of the boundary of that shape. Hence, we have calculated circumference of the semicircle. Also, when we find the circumference of the semicircle, it does not include the length of its diameter.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




