
The change in the value of g at a height h above as the surface of the earth is the same at a depth d below the surface of the earth. When both d and h are much smaller than the radius of earth, then which one of the following is correct?
Answer
445.7k+ views
Hint: We will take the help of the formulas used for finding the value of gravity above and below earth’s surface along with the defined parameters. We will apply the condition discussed in the question about the same value of h and d where h is the height of the object to be calculated and d is the depth. Both h and d are very less than the radius of earth.
Formula used:
Complete step by step answer:
For this we are going to consider the following two diagrams which show earth’s variations with respect to gravity and height, explained in the figure.
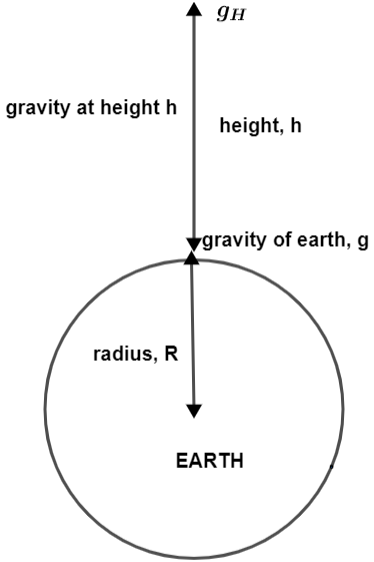
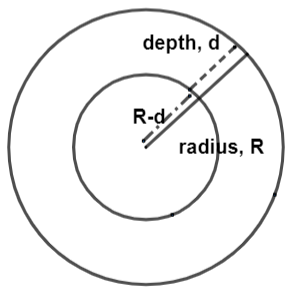
Suppose that the acceleration due to gravity at height h is represented as
Aas the formula of gravitational force at height h is
So, the correct answer is “Option B”.
Note:
The height above the earth’s surface has been selected to find gravity at that same height from the surface. This is useful in finding the value of gravity at that level exerted by any object that has been taken as an object. If we consider a depth inside the earth’s surface then, we will find the presence of gravity there also but the difference will be the slight change in the value which can be calculated by the given formula. Here, h << R because we are taking the height of an object above the surface of earth to be very less than the radius of the earth. Similarly, we have d << R. As by the statement in the question informing the value change of g at some height h taken above earth’s surface is the equal to the value change of gravity at some depth d downwards the earth’s surface.
Formula used:
Complete step by step answer:
For this we are going to consider the following two diagrams which show earth’s variations with respect to gravity and height, explained in the figure.


Suppose that the acceleration due to gravity at height h is represented as
Aas the formula of gravitational force at height h is
So, the correct answer is “Option B”.
Note:
The height above the earth’s surface has been selected to find gravity at that same height from the surface. This is useful in finding the value of gravity at that level exerted by any object that has been taken as an object. If we consider a depth inside the earth’s surface then, we will find the presence of gravity there also but the difference will be the slight change in the value which can be calculated by the given formula. Here, h << R because we are taking the height of an object above the surface of earth to be very less than the radius of the earth. Similarly, we have d << R. As by the statement in the question informing the value change of g at some height h taken above earth’s surface is the equal to the value change of gravity at some depth d downwards the earth’s surface.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




