
The circum radius of the triangle formed by the points
A.
B.14
C.
D.0
Answer
484.8k+ views
Hint: We find the lengths of the sides using the distance formula between any two points with coordinates
Complete step by step answer:
We know that the distance
We also the circumcircle of a triangle is a circle that passes through the vertices of the circles. The centre of the circumcircle is called circumcentre and the radius of the circum circle is called circumradius. The length of the circumradius with length of the circle is given by
If the triangle is equilateral triangle
We are given the coordinates of the vertices of the triangle
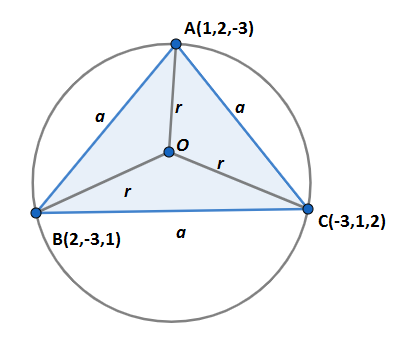
So we first find lengths of the sides using the distance formula. We find AB whose endpoints are
We find AC whose endpoints are
We find BC whose endpoints are
We see that
So, the correct answer is “Option A”.
Note: We note that distance is always a positive quantity and hence we have rejected negative square roots. We can alternatively solve by finding the circumcentre O and distance between O to any vertex. We find the circumcenter as a point of intersection lines that passes through midpoints of sides and lying in the plane of the triangle. The circumcentre is given by
Complete step by step answer:
We know that the distance
We also the circumcircle of a triangle is a circle that passes through the vertices of the circles. The centre of the circumcircle is called circumcentre and the radius of the circum circle is called circumradius. The length of the circumradius with length of the circle is given by
If the triangle is equilateral triangle
We are given the coordinates of the vertices of the triangle

So we first find lengths of the sides using the distance formula. We find AB whose endpoints are
We find AC whose endpoints are
We find BC whose endpoints are
We see that
So, the correct answer is “Option A”.
Note: We note that distance is always a positive quantity and hence we have rejected negative square roots. We can alternatively solve by finding the circumcentre O and distance between O to any vertex. We find the circumcenter as a point of intersection lines that passes through midpoints of sides and lying in the plane of the triangle. The circumcentre is given by
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




