
The circumcentre of a triangle lies at the origin and it’s centroid is the midpoint of the line segment joining the points
A)
B)
C)
D)
Answer
485.1k+ views
Hint: We know that circumcentre is the intersection point of all the perpendicular bisectors and centroid is intersection point of all the medians of the given triangle and orthocenter is the intersection of all angular bisectors of the given triangle. These points i.e. circumcentre, centroid and orthocenter are always collinear.
Complete step by step answer:
Given that the circumcentre(C) of triangle XYZ is origin which implies C = (0,0)
Also Given that the centroid (G) is the midpoint of the line segment joining the points E
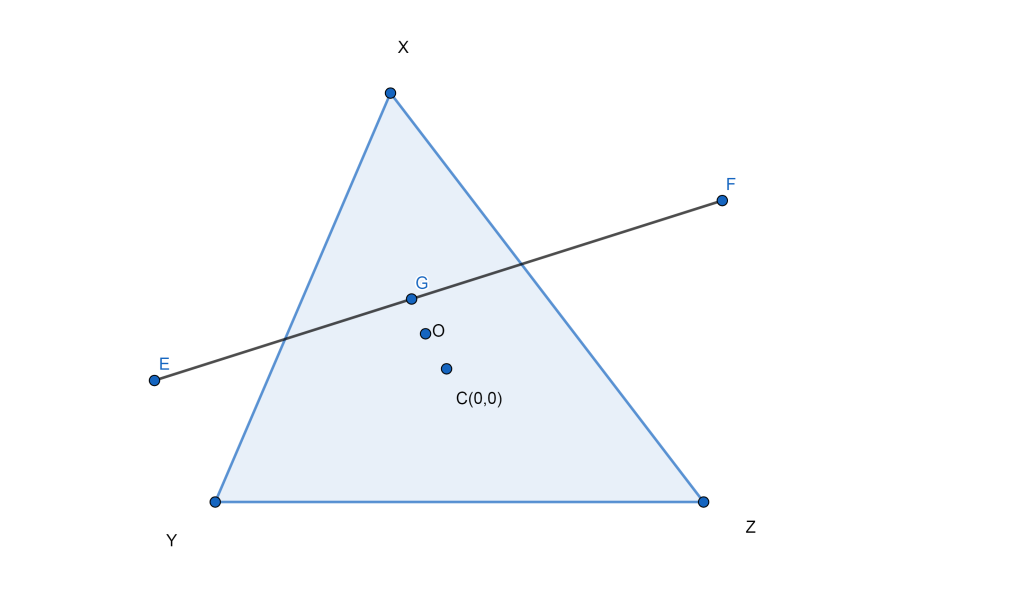
We know that midpoint of any two points(a,b) and (c,d) is (
which implies the centroid (G) = (
[We already know that
As we know that centroid , circumcentre , orthocenter are collinear , we can find the line that passes through these three points even when we know any of these two points.
So, now we will find the line passing through G and C so that the orthocenter lies on it.
We know that the equation of line that passes through (a,b) and (c,d) is
which implies the line passing through G (
Which implies
Which implies
That is also equal to
Therefore the orthocenter of this triangle lies on the line
So, the correct answer is “Option D”.
Note:
Go through the properties of all the circles and centres corresponding to the triangle that is orthocenter , centroid, circumcenter , incenter and find the ratios through which one center cuts the other .Also check the collinearities between them. Do not draw the graph whenever you see a question related to these centers. First read the total question and then solve the problem.
Complete step by step answer:
Given that the circumcentre(C) of triangle XYZ is origin which implies C = (0,0)
Also Given that the centroid (G) is the midpoint of the line segment joining the points E

We know that midpoint of any two points(a,b) and (c,d) is (
which implies the centroid (G) = (
[We already know that
As we know that centroid , circumcentre , orthocenter are collinear , we can find the line that passes through these three points even when we know any of these two points.
So, now we will find the line passing through G and C so that the orthocenter lies on it.
We know that the equation of line that passes through (a,b) and (c,d) is
which implies the line passing through G (
Which implies
Which implies
That is also equal to
Therefore the orthocenter of this triangle lies on the line
So, the correct answer is “Option D”.
Note:
Go through the properties of all the circles and centres corresponding to the triangle that is orthocenter , centroid, circumcenter , incenter and find the ratios through which one center cuts the other .Also check the collinearities between them. Do not draw the graph whenever you see a question related to these centers. First read the total question and then solve the problem.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

The plastids which are coloured green and colourless class 11 biology CBSE

Earth rotates in which direction A East to west B West class 11 physics CBSE




