
The coordinates for a rhombus are given as
Answer
455.4k+ views
Hint: The Midpoint Formula works precisely the same way as If you would like to search out the purpose that's exactly halfway between two given points, just average the x-values and therefore the y-values.
Complete step-by-step solution:
Let the coordinates of a rhombus as
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
It can be seen that
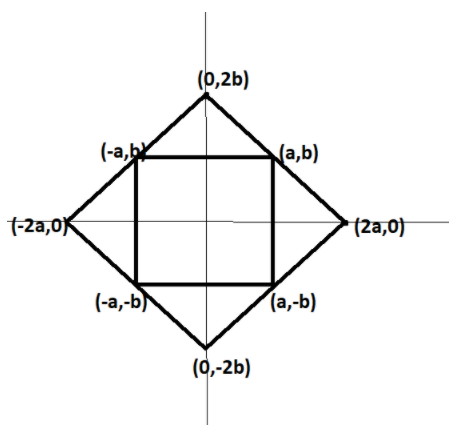
Hence, the mid points of the rhombus form the rectangle.
Note: Sometimes you would like to seek out the purpose that's exactly midway between two other points. For example, you may find a line that bisects (divides into two equal halves) a given line segment. This middle point is named the "midpoint". The concept doesn't come up often, but the Formula is sort of simple and obvious, so you must easily be able to recall it for later.
Complete step-by-step solution:
Let the coordinates of a rhombus as
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
Let the midpoint of
Therefore , coordinates are given as ,
It can be seen that

Hence, the mid points of the rhombus form the rectangle.
Note: Sometimes you would like to seek out the purpose that's exactly midway between two other points. For example, you may find a line that bisects (divides into two equal halves) a given line segment. This middle point is named the "midpoint". The concept doesn't come up often, but the Formula is sort of simple and obvious, so you must easily be able to recall it for later.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




