
The correct geometry and hybridization of
A.Octahedral,
B.Trigonal bipyramidal,
C.Planar triangle,
D.Square planar,
Answer
499.2k+ views
Hint: To answer this question, you should recall that xenon is a noble gas and when bonding it will have the tendency to use all the eight electrons in the outermost shell to form bond pairs. We know fluorine is a halogen and can form one sigma bond with the central atom. Now, use this information to answer the question.
Complete step by step answer:
It is evident from the formula
As we know that fluorine can form a single sigma bond, it will be bonded to
So, the hybridization of this molecule will be
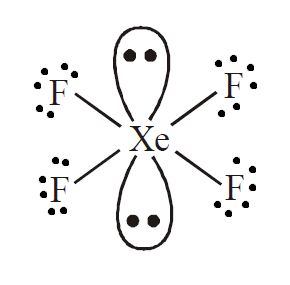
Therefore, we can conclude that the correct answer to this question is option D.
Note:
Even if you are not able to calculate the hybridisation using the above-mentioned, you can find the hybridization
The value of
Now, to determine the hybridization of
Here, the total valence electrons,
Substituting in the formula we get,
Thus, hybridization of
Complete step by step answer:
It is evident from the formula
As we know that fluorine can form a single sigma bond, it will be bonded to
So, the hybridization of this molecule will be

Therefore, we can conclude that the correct answer to this question is option D.
Note:
Even if you are not able to calculate the hybridisation using the above-mentioned, you can find the hybridization
The value of
Now, to determine the hybridization of
Here, the total valence electrons,
Substituting in the formula we get,
Thus, hybridization of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




