Question

The decimal expansion of $\sqrt 2 $ is:
A. Finite decimal
B. 1.4121
C. Non – terminating recurring
D. Non – terminating non-recurring

Answer 1

Hint: First try to find the value of the given square root using the long division method. The value obtained can be used to easily choose the correct option.

Complete Step-by-Step solution:
First, we need to find the value of $\sqrt 2 $.
One of the methods of finding the square root is the long division method.
Steps involved in a long division method are explained below:
Step 1. The digits of the number are needed to be grouped in pairs starting from the unit's place before the decimal. Pair the digits starting from the digit after decimal.
Step 2. Choose the largest number whose square is equal to or just less than the first pair from right. Take this number as divisor and the quotient.
Step 3. Subtract the product of divisor and quotient from the first pair and group the remainder with the next pair which forms the new dividend.
Step 4. Now the new divisor is chosen by raking two times the quotient and succeeding it with a suitable digit which is also taken as the quotient chosen in such a way that the product of divisor and quotient is equal or less than the dividend.
Step 5. Repeat steps 2 to 4 till all the pairs are exhausted.
Step 6. If the remainder is not zero at the end of the pairs, add decimal after the dividend and repeat the steps 2 to 4 by adding a pair of zeros after the decimal till the desired value is obtained.
The above method is applied to 2 to calculate the value of $\sqrt 2 $.

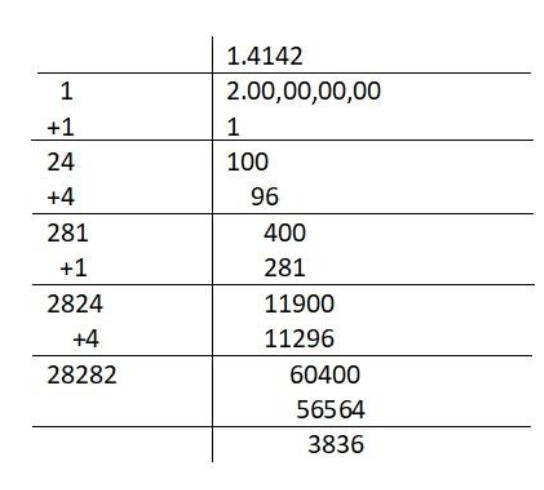
The decimal places in the quotient obtained by the long division method goes on increasing as the zeros are added in the dividend according to steps 6 in the above explained method.
Hence the quotient has non-terminating value in decimal places.
Also, it is to be noted that the decimal digits in the quotients are non-repeating or non-recurring.
Hence the decimal expansion of $\sqrt 2 $ is non – terminating non-recurring.
Therefore, option (D). Non – terminating non-recurring is the correct answer.

Note: The above method of long division to calculate the square roots should be kept in mind in problems like above. Also, if a square root is not a perfect square, then it is considered an irrational number. An irrational number is a number that cannot be expressed as a fraction. Irrational numbers have decimal expansions that are non-terminating and non-recurring.