
The diagonals of a parallelogram ABCD intersect at O. A line through O intersects AB at X and DC at Y. Prove that
Answer
437.1k+ views
Hint: We will first of all construct a neat and clean diagram with proper labels to proceed in our question. Now, to prove
Complete step by step answer:
It has been given that the ends of the parallelogram are A, B, C and D respectively. Also, a line through O intersects AB at X and DC at Y. Now, we have enough information required for the construction of our parallelogram. This parallelogram can be constructed as follows:
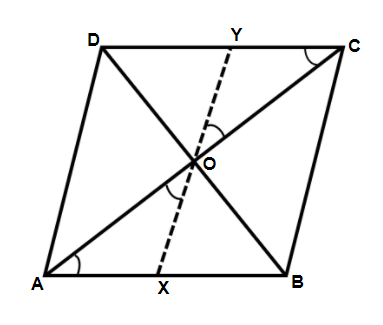
Now, we can see in the above figure that, to prove
In the triangles OAX and OCY, we have:
This is true by the property of opposite angles subtended between two-line pairs.
Now, we have:
This is true because diagonals of a parallelogram bisect each other.
And, at last we have:
This is true by the property of alternate interior angles of two parallel lines.
Therefore, using these three equations, we can apply the angle-side-angle (ASA) postulate of congruence. Thus, we can write:
Therefore, now we can say that:
Hence,
Note: Proper construction and labelling of diagrams is very important when going for a proof in mathematics. It helps us see things and data more clearly. So, the diagram should always be neat. Also, one should be very careful in using the correct postulate while proving congruence of two triangles.
Complete step by step answer:
It has been given that the ends of the parallelogram are A, B, C and D respectively. Also, a line through O intersects AB at X and DC at Y. Now, we have enough information required for the construction of our parallelogram. This parallelogram can be constructed as follows:

Now, we can see in the above figure that, to prove
In the triangles OAX and OCY, we have:
This is true by the property of opposite angles subtended between two-line pairs.
Now, we have:
This is true because diagonals of a parallelogram bisect each other.
And, at last we have:
This is true by the property of alternate interior angles of two parallel lines.
Therefore, using these three equations, we can apply the angle-side-angle (ASA) postulate of congruence. Thus, we can write:
Therefore, now we can say that:
Hence,
Note: Proper construction and labelling of diagrams is very important when going for a proof in mathematics. It helps us see things and data more clearly. So, the diagram should always be neat. Also, one should be very careful in using the correct postulate while proving congruence of two triangles.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




