
The diagonals of a parallelogram
A. Rectangle
B. Square
C. Cyclic quadrilateral
D. Rhombus
Answer
525.6k+ views
1 likes
Hint: Take a slope of given two equations and multiply it. If the product is
Complete step by step answer:
So here we have a parallelogram
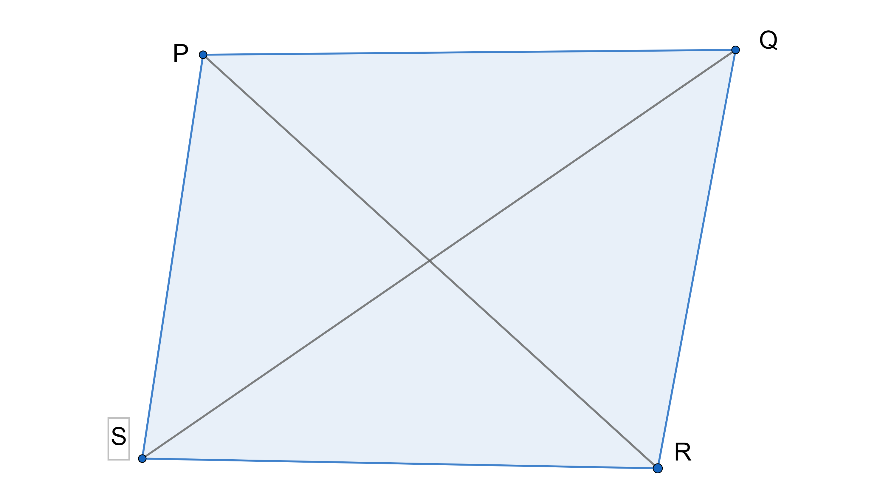
So we can see the parallelogram above
Let
So now we will take the slope of line
So to find out slope we should convert
So converting we get
So let slope of line
So
So now we will take the slope of line
So to find out slope we should convert
So converting we get
So let slope of line
So
So we know the property that if two slopes of two different lines are multiplied and we get the final value as
So let us see for
So we can see that the lines are perpendicular.
So
i.e. we can say that
So here we get two options, that is it might be rhombus or it might be square.
Because diagonals of rhombus and square bisect each other at right angles.
Squares are a special case of parallelograms itself
Since it is given that
So we know that the angle between any two sides of a parallelogram and rhombus is not equal to
So incase of square the angle between any two sides is
Squares are a special case of parallelograms itself.
So the parallelogram can be square or a rhombus.
Option(B) and Option (D) are correct answers.
Note: So just keep in mind that you should know properties of square, rhombus, parallelogram etc.
Sometimes jumbling occurs while converting the equation into
Complete step by step answer:
So here we have a parallelogram

So we can see the parallelogram above
Let
So now we will take the slope of line
So to find out slope we should convert
So converting we get
So let slope of line
So
So now we will take the slope of line
So to find out slope we should convert
So converting we get
So let slope of line
So
So we know the property that if two slopes of two different lines are multiplied and we get the final value as
So let us see for
So we can see that the lines are perpendicular.
So
i.e. we can say that
So here we get two options, that is it might be rhombus or it might be square.
Because diagonals of rhombus and square bisect each other at right angles.
Squares are a special case of parallelograms itself
Since it is given that
So we know that the angle between any two sides of a parallelogram and rhombus is not equal to
So incase of square the angle between any two sides is
Squares are a special case of parallelograms itself.
So the parallelogram can be square or a rhombus.
Option(B) and Option (D) are correct answers.
Note: So just keep in mind that you should know properties of square, rhombus, parallelogram etc.
Sometimes jumbling occurs while converting the equation into
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




