
The diagram alongside shows a uniform meter rule of weight
(a) The value of
(b) The resultant moment and its direction if the weight
(c) The position of another weight of
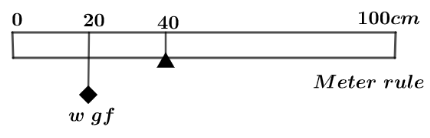

Answer
431.7k+ views
Hint: In order to solve this question, we will use the concept of principle of moments. Which states that in equilibrium state of forces the clockwise moment about a point is always equal to its anticlockwise moment. Moment is the product of force and perpendicular stable distance point of suspension.
Complete step by step answer:
(a) Let us first find the clockwise moment about weight
Is given by,
Clockwise moment
Now, an anticlockwise moment is given as,
Anticlockwise moment
Comparing both equations
Hence, the unknown weight is
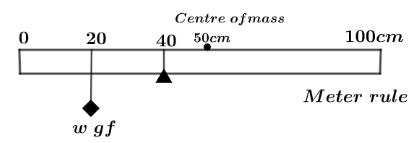
(b) If weight is now moved to the point
Moment
Moment
(c) Let the position of new weight
Since, we have anticlockwise Moment
And, calculating clockwise moment as:
Equating both equations
Hence, the new weight
Note:Principle of moment is based upon the concept that a weight tends to produce an anticlockwise moment about its equilibrium point this makes system unstable and hence if it’s placed in such a point where its clockwise moment will just equals to the anticlockwise moment, system will remain in equilibrium as long as the positions of weights don’t changes.
Complete step by step answer:
(a) Let us first find the clockwise moment about weight

Is given by,
Clockwise moment
Now, an anticlockwise moment is given as,
Anticlockwise moment
Comparing both equations
Hence, the unknown weight is
(b) If weight is now moved to the point
Moment
Moment
(c) Let the position of new weight
Since, we have anticlockwise Moment
And, calculating clockwise moment as:
Equating both equations
Hence, the new weight
Note:Principle of moment is based upon the concept that a weight tends to produce an anticlockwise moment about its equilibrium point this makes system unstable and hence if it’s placed in such a point where its clockwise moment will just equals to the anticlockwise moment, system will remain in equilibrium as long as the positions of weights don’t changes.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




