
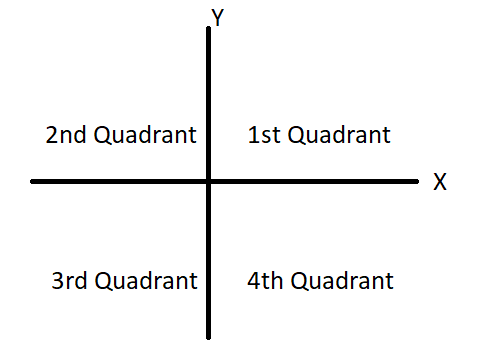
The diagram shows all the four quadrants in a Cartesian Plane.
Which of the following points below lies in

Answer
429.3k+ views
Hint: We are given four different points out of which we have to identify which point lies in the third quadrant. If the two coordinates are in the form of
Complete step by step solution:
The Cartesian plane creates four quadrants (I, II, III, IV).
We are given the diagram that shows all the four quadrants in a Cartesian Plane.
The points lies in
The coordinate point
Following points should be kept in mind about the points falling in the quadrants:
Quadrant I= Both the
Quadrant II=Coordinate
Quadrant III= Both the
Quadrant IV=Coordinate
It can be shown diagrammatically as follows:
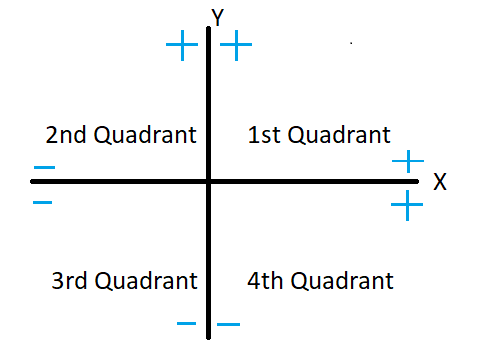
It shows the positive and negative sign of coordinates falling in the four quadrants.
Now we can solve the question:
Out of all the points, only point
Hence,
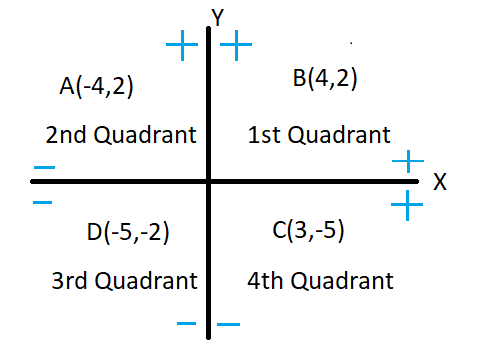
The points given in the question can be plotted on the Cartesian plane as follows:
Note:
The Cartesian plane is defined as a two-dimensional coordinate plane, which is formed by the intersection of the
The
Complete step by step solution:
The Cartesian plane creates four quadrants (I, II, III, IV).
We are given the diagram that shows all the four quadrants in a Cartesian Plane.
The points lies in

The coordinate point
Following points should be kept in mind about the points falling in the quadrants:
Quadrant I= Both the
Quadrant II=Coordinate
Quadrant III= Both the
Quadrant IV=Coordinate
It can be shown diagrammatically as follows:

It shows the positive and negative sign of coordinates falling in the four quadrants.
Now we can solve the question:
Out of all the points, only point
Hence,
The points given in the question can be plotted on the Cartesian plane as follows:

Note:
The Cartesian plane is defined as a two-dimensional coordinate plane, which is formed by the intersection of the
The
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE

Write an essay in about 150 200 words on the following class 9 english CBSE

Differentiate between parenchyma collenchyma and sclerenchyma class 9 biology CBSE

The Fundamental Right which cannot be suspended even class 9 social science CBSE

What were the main changes brought about by the Bolsheviks class 9 social science CBSE




